A smaller version of this demonstration, shown below, is available for use in classrooms outside the Broida lecture hall building.
With this demonstration, you can illustrate the concepts of tension, and balanced and unbalanced forces.
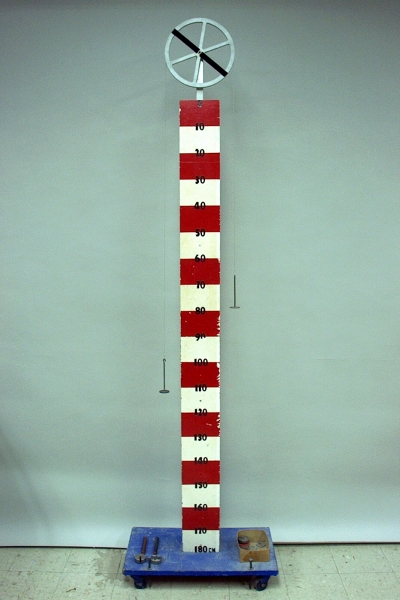
A mass hanger hangs from each side of a large pulley. When the masses on each side equal (as in the photograph), the hangers do not move. When you hang different masses from each side, one accelerates downwards, and the other upwards, at a rate that depends on the difference between the masses, hence the connection to Newton’s second law of motion, which (as quoted in Sears, Francis Weston and Zemansky, Mark W. College Physics, Third Edition (Reading, Massachusetts: Addison-Wesley Publishing Company, 1960), p. 78) states, The change of motion is proportional to the motive force impressed; and is made in the direction of the straight line in which that force is impressed. If we take “change of motion” to mean the rate of change of velocity, or an acceleration, and remember that an object’s inertia is proportional to its mass, we end up with the familiar F = ma.
Each hanger has a mass of 50 g. The box sitting to the right on the base of the apparatus contains a variety of slugs of different mass, which you can set on the hangers to adjust their masses as desired. Depending on how quantitative you would like to make the demonstration, you can ignore the mass of the pulley and the friction in the pivot, or you can take them into account by timing the descent of the falling mass, finding the acceleration, and then noting by how much it differs from what you would expect for a massless pulley and frictionless pivot. Should you wish to change the moment of inertia of the pulley by a known amount, two 200-g masses and two 13-cm-long, 145-g rods, shown on the left of the base of the apparatus, are available for attachment to two of the spokes of the pulley, by means of Velcro® strips attached to them. The radius of the groove in which the string runs on the pulley is 12.25 cm.
The two main questions regarding this system are what the accelerations are of the two masses (which have to be equal and opposite, unless the string breaks), and what the tension is in the string. We assume that the tension is the same for the whole length of the string; that is, that it is unaffected by friction between the string and the groove of the pulley. Let us refer to the mass on the left as m1, and the one on the right as m2. If we ignore the mass of the pulley and the friction in its bearing, we can write two equations: T - m1g = m1a, and T - m2g = -m2a, where T is the tension in the string. Combining these equations to eliminate T yields a = [(m2 - m1)/(m1 + m2)]g. Substituting for a in either of the first two equations gives T = [2m1m2/(m1 + m2)]g. We can see from these equations that if the two masses are equal, a = 0, that is, the masses remain stationary, and T = mg. Each mass exerts a downward force of mg on the string, which, in turn, exerts an upward force on each of mg. The tensions on both sides of the pulley are equal. The pulley pivot and stand, however, must provide 2T, plus the weight of the pulley, to support the pulley and the two masses.
For an example of what happens with unequal masses hung from either side, we can set m2 = m1/2. This gives a = [(m1/2 - m1)/(m1 + m1/2)]g, or (-m1/2)/(3m1/2)]g = (1/3)g, or -g/3. The tension is T = [2m1(m1/2)/(m1 + m1/2)]g, or T = (2m1/3)g. So the acceleration (of m1 downward and m2 upward) is one-third the acceleration of gravity, and the tension in the string is two-thirds the weight of m1, or one-and-one-third times the weight of m2, intermediate between the weights of the individual masses.
If the masses are greatly unequal, say m1 ≫ m2, then a ≈ -g, and T ≈ 2m2g. m1 is almost in free fall, and the tension is almost twice what it would be in the static situation when both masses equal m2.
To take into account the inertia of the pulley, still neglecting friction, we can take the tangential force on the pulley as m1g - m2g, or F = (m1 - m2)g. This force, however, has to accelerate not only the pulley, but the masses, so we have F = (m1 + m2)a + Frot. You can measure a by timing the fall of one of the masses from where it starts to some arbitrary distance below the starting point. The average velocity is the fall distance divided by the time, the final velocity is twice this, and the acceleration is the final velocity divided by the fall time. The angular acceleration, α, of the pulley is a/r, the linear acceleration divided by the radius of the pulley, which for the apparatus above is 0.122 m. Once you have calcluated F and measured a, you can find Frot = F - (m1 + m2)a. The torque on the pulley is rFrot, which also equals Iα, where I is the moment of inertia of the pulley. From this, we have I = rFrot/α. If you knew the actual moment of inertia of the pulley, you could calculate Frot from it and the measured α. The difference between F and the sum (m1 + m2)a + Frot would give the frictional force. Since the friction is probably no more than a few percent, however, the calculated moment should be fairly close.
Alternatively, you could increase the moment of inertia by a known amount, by adding either the 200-g masses or the 145-g rods (which, essentially, should behave as one 26-cm rod weighing 290 g). In this case, rFrot = Iα + I0α, where I0 is the moment of inertia of the pulley before you added the masses. As mentioned above, without knowing this moment of inertia, it is not possible to determine the effect of friction in the pulley bearing.
As noted above, a smaller, portable version of this demonstration is available:
This apparatus uses two 2-inch-diameter pulleys in place of a single large pulley, but the physics is identical to that in the larger system. (The diameter of the groove in each pulley is about 1.88″.) It is shown with a large stand base and a 3/4-inch rod. It is possible to assemble it with a 1/2-inch rod and a medium stand base, but the larger assembly is more stable, and probably still light enough to carry.