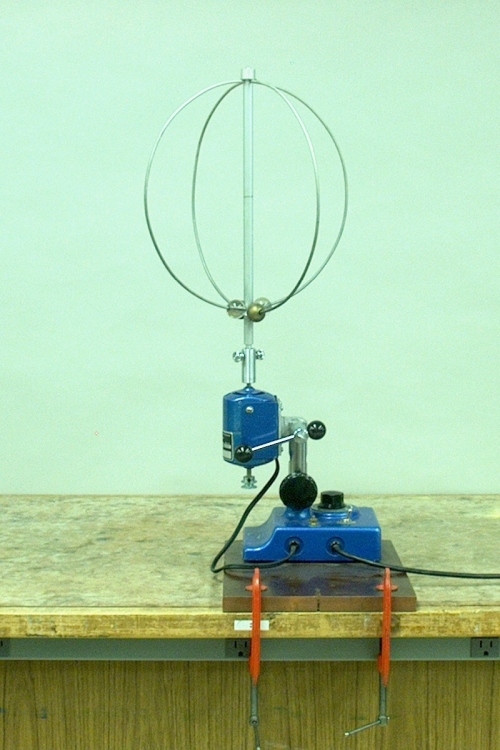
Two brass balls and two plastic balls are able to slide freely on two vertical hoops, which are mounted to a central spindle that is attached to a variable-speed motor. When you turn on the motor, the balls begin to slide outward. As you increase the speed of the motor, the balls move further out, and further up, the hoops.
Shown in the photograph above is a variable-speed motor, to whose output shaft is attached a pair of hoops on which two brass balls and two plastic balls are set. All the balls have the same diameter, so the brass balls have greater mass than the plastic balls. Each ball has a hole drilled through its center, and the hoop on which it rides passes through the hole. When you turn the motor on, the hoops begin to rotate about the spindle, causing the balls to revolve about the central axis. As the previous demonstrations in this chapter show, at any instant, in order to keep the balls moving in a circle instead of straight lines, the hoops must provide a sideways force on them, accelerating them toward the center of the circle – a centripetal force. This force is F = mv2/r, where m is the mass of the ball, v is its tangential velocity, and r is the radius of the circle in which it travels. (See demonstration 16.03 -- Ball on turntable.) The tangential velocity, v, equals ωr, where ω is the angular velocity of the spindle, hoops and balls. We can express the centripetal force as F = mω2r. The hoops must also support the balls against gravity. Wherever a ball is on one of the hoops, the hoop exerts a normal force, N, on it, that is, a force directed inward along a radius of the hoop (perpendicular to the tangent to the hoop). This normal force has two components, which provide the centripetal force and balance gravity. These are
N sin θ = mω2r and N cos θ = mg
where θ is the angle between vertical and the radius on which the ball lies. If we divide the first equation by the second, we obtain
tan θ = ω2r/g
Since r, the radius of the circle in which the ball travels, equals R sin θ, where R is the radius of the hoop, we have
tan θ = ω2R sin θ/g
which gives
cos θ = g/ω2R
We see that for any particular rotational speed, both the brass balls and the plastic balls reach the same level, which increases toward a maximum (just below the horizontal) as the speed increases.
If we wish to consider friction, for the case in which the balls are rising as the speed increases, the friction is F = μN, and it acts downward along the tangent to the hoop where the ball sits. Its two components are
μN cos θ (horizontal) and -μN sin θ (vertical)
Adding these to the first pair of equations above gives
mω2r = N sin θ + μN cos θ and mg = N cos θ - μN sin θ
Combining these gives
ω2 = [(g/r)(sin θ + μ cos θ)]/(cos θ - μ sin θ)
or
ω2 = [(g/r)(tan θ + μ)]/(1 - μ tan θ)
If we consider the case in which the balls are sliding down, friction acts in the opposite direction, which reverses the sign of its two components, and this equation becomes
ω2 = [(g/r)(tan θ - μ)]/(1 + μ tan θ)
We can rearrange the equation before this to get
tan θ = [(ω2r/g) - μ]/[1 + (ω2r/g)μ]
As noted above, r = R sin θ, so this becomes
tan θ = [(ω2R sin θ/g) - μ]/[1 + (ω2R sin θ/g)μ]
Without friction, this reduces to the exression given above (cos θ = g/ω2R). Suffice it to say that without friction, at a given angular speed, both sets of balls rise to the same height, independent of mass. If the friction for both sets of balls is similar, or the contribution of friction is small, the difference in height to which the two sets of balls rise is small.
References:
1) Sears, Francis Weston and Zemansky, Mark W. College Physics, Third Edition (Reading, Massachusetts: Addison-Wesley Publishing Company, Inc., 1960), p. 110.
2) Miller, Julius Sumner. Demonstrations in Physics (Sydney, Australia: Ure Smith Pty Limited, 1969), pp.68-69.