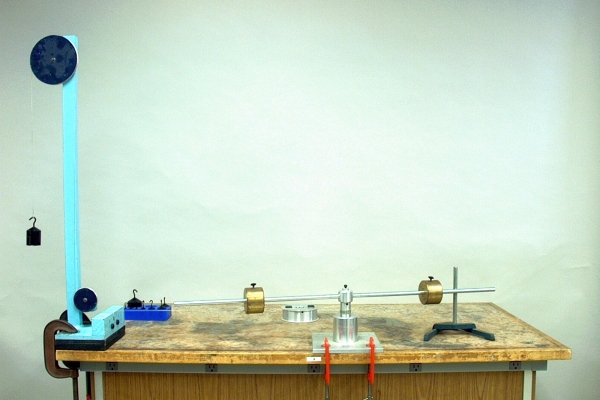
A horizontal rod carrying a mass on each side is attached at its center to a vertical spindle. A string attached to the spindle passes around two pulleys to a hanging mass, which, when the string is wound around the spindle, causes the rod to rotate as it falls. You wind the string around the spindle by rotating the rod by hand. You can vary the moment of inertia of the horizontal rod by changing the position of the two brass masses, which are fastened by means of thumbscrews. A collar, shown at the rear of the table, allows you to increase the diameter of the spindle where the string wraps, should you wish to do so. You can hang different masses from the end of the string, to change the applied force. A rod on a stand base acts as a brake to keep the apparatus from unwinding until you wish to use it.

The masses are 1.89 kg (4.16 lb), and the rod has a line every two inches (5.1 cm) from the center. The masses themselves are two inches wide, so you can place them with a mark exactly at each side (so odd inch distances from the center), or with a mark at the center (by leaving equal space between the mass and the mark at either side; even inch distances from the center). The spindle diameter is 1.25″, and the collar increases it to 5.0″, for a radius of 0.625″ (1.59 cm) and 2.5″ (9.4 cm), respectively.

Demonstration 28.03 -- Mounted bicycle wheel, illustrates the variables of position, velocity and acceleration for rotational motion, which are analogous to those of rectilinear motion, and gives the corresponding equations of motion. Similarly, whereas objects resist changes in their straight-line motion according to their mass, this demonstration shows the analogous resistance of an object to change in rotational motion according to its moment of inertia.

The pages for the earlier demonstrations in this chapter present torque as r × F, the cross product of the displacement of the point of application of a force from the axis of motion, and the force, where the torque is opposing another torque, as in 28.06 -- Lever arm, in which gravity exerts both torques, 28.12 -- Wrenches, screwdrivers, where friction and tension oppose torque you apply via a tool, 28.15 -- Coconut cracker, where compressive forces in the coconut oppose the torque you apply to the cracker, or 28.18 -- Open door to demonstration preparation area, where a door closer opposes torque that you apply to the door. Here we consider a single torque acting on an object, and the resulting acceleration of the object (ignoring the translational force exerted by the tension in the string, and the friction force exerted by the clamps on the spindle base, which opposes it). We also examine how the acceleration due to a particular torque changes with the moment of inertia of the object being accelerated.

All points on a rigid body rotating about a particular fixed axis have the same angular speed, ω, but an instantaneous linear speed, v = ωr, where r is the distance of a particular point from the axis of rotation. Such a point has a kinetic energy (1/2)mv² = (1/2)mr²ω². For the whole body, the kinetic energy is the sum of the kinetic energies of all of the points, or K = (1/2)(m₁r₁² + m₂r₂² + · · ·)ω² = (1/2)(Σ m_ir_i²)ω². We can denote the sum of the products of the masses and the squares of their distances from the axis I, or I = Σ m_ir_i². This quantity is known as the rotational inertia or moment of inertia of the body with respect to the axis about which it is rotating. Because the elements of the sum contain products of mr², we can see that the moment of inertia of an object depends both on the orientation of the axis about which it rotates, and on the object’s shape and its distribution of mass. Its units, mass·length², are usually expressed as kg·m², g·cm² or slug·feet². Putting the rotational kinetic energy in terms of I, then, we have K = (1/2)Iω², which is analogous to translational kinetic energy, and in which ω is analogous to v, linear speed, and I is analogous to m, the linear inertia. As noted above, just as an object’s resistance to changes in its straight-line motion goes in proportion to its mass, its resistance to changing its rotational speed about a particular axis is proportional to I, its moment of inertia about that axis. We now wish to find the rotational analogue of F = ma.

If we apply a force, F, to some point on a body that can rotate, in a small time interval, dt, the point moves a distance ds = r dθ, and the work done during this interval is dW = F · ds = F cos φ ds = (F cos φ)(r dθ). (F cos φ) is the component of F in the direction of ds, that is, perpendicular to r, or tangent to the circle of motion. The magnitude of the instantaneous torque exerted by F is (F cos θ) r, so we have dW = τ dθ, which is equivalent to the expression for work done in a straight line, dW = F dx. The rate at which work is being done on the rotating body is dW/dt = τ dθ/dt = τω. This must equal the change in kinetic energy of the body, which is d/dt ((1/2)Iω²). Since we are dealing with a rigid body rotating about a fixed axis, I is constant, and we have d/dt ((1/2)Iω²) = (1/2)I d/dt (ω²) = Iω (dω/dt) = Iωα. So we have τω = Iωα, or τ = Iα.

In this demonstration, F is provided by the tension, T, in the string, which is provided by gravity acting on the hanging mass. When the apparatus is being held still, and the mass is not falling, T merely equals mg, where m is the mass of the hanging mass. As soon as you release the apparatus, though, and the mass begins to fall and the apparatus begins to rotate, the tension must be less than mg, or mg - T = ma, which gives T = mg - ma. If we use r for the radius of the spindle, Tr = Iα, and since α = a/r, T = Ia/r². Solving these equations gives a = mg/(m + (I/r²)), and T = mgI/(mr² + I). So we see that for a given hanging mass, the greater the moment of inertia of our apparatus, the more slowly the hanging mass accelerates downwards, the smaller the angular acceleration of the apparatus (= a/r), and the closer the tension is to mg. Conversely, the smaller the moment of inertia of the apparatus, the greater the downward acceleration of the hanging mass, the greater the angular acceleration of the apparatus, and the smaller the tension in the string, down to a limiting value of T = g(I/r²) (when m >> I). Also, the greater the hanging mass, the greater its downward acceleration, and thus the angular acceleration of the apparatus. As noted above, the spindle radius without the collar is 1.59 cm, and with the collar it is 9.4 cm, so for a given tension, adding the collar increases the torque by a factor of 5.9.

For our apparatus, the moment of inertia is just I = ((1.89 kg)R₁² + (1.89 kg)R₂²), where 1.89 kg is the mass of each of the two brass slugs, and R₁ and R₂ are the distances at which you set them from the center of the spindle. If you place them at the same distance from the center, this becomes I = (3.78 kg) R². We have neglected the mass of the rod and spindle themselves, which is fairly small compared to the masses of the brass slugs. Still, the closer the slugs are to the center, the smaller the moment of inertia, and the farther they are from the center, the greater the moment of inertia. The rod and spindle add only a small, constant contribution to the moment of inertia of the apparatus.

References:

1) Resnick, Robert and Halliday, David. Physics, Part One, Third Edition (New York: John Wiley and Sons, 1977), pp. 237, 243-6.
2) Sears, Francis Weston and Zemansky, Mark W. College Physics, Third Edition (Reading, Massachusetts: Addison-Wesley Publishing Company, Inc., 1960), pp. 180, 184-5.