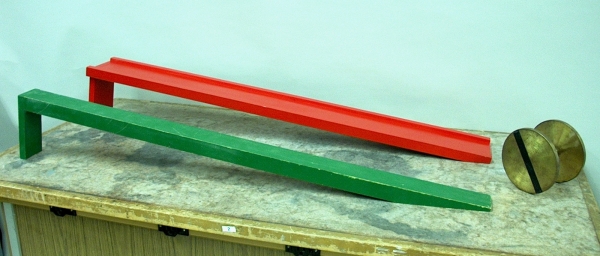
Place the yo-yo at the top of the green ramp. (The spindle rests on the ramp, and the two discs straddle it.) Now release it. It rolls slowly down the ramp, until the edges of the discs touch the table, at which point it continues at a much higher speed. CAUTION: For this reason, you should perform this demonstration on the long, main demonstration table. This way, there will be greater distance to show the yo-yo moving at the new, higher speed, and you will have more time to get ready to catch the yo-yo before it rolls off the end of the table. If it falls off the table, chances are very good that it will break. If you now place the yo-yo on the red ramp (on which the discs fit between the rails at the edges of the ramp), starting from the same height as on the green ramp (marked by an arrow just above halfway down the ramp), it now accelerates much faster, and when it reaches the bottom of the ramp, continues on at the same speed it had just when it reached the end of the ramp. This speed, however, is about √(1/3) times (~0.58) the speed it had when it sped away from the green ramp.

The yo-yo comprises two brass discs of radius 3.00″ (76.2 mm), each having a mass of approximately 1.4 kg (their total mass is 2.774 kg), and a spindle of radius 0.249″ (6.32 mm) and mass 0.096 kg. If we treat the yo-yo as a pair of discs of uniform mass, this leads to an error of about 3 percent in the moment of inertia, but greatly simplifies our analyses below (and introduces a smaller overall error in our calculations for velocity and time, since it ends up under a radical sign). The green ramp has a height of 5-7/16″ (0.139 m), which puts the edges of the yo-yo 4-3/16″ (0.106 m) above the table. The distance the yo-yo travels along the ramp until its edges make contact with the table is about 30.0″ (0.762 m). The ramp angle is approximately 8 degrees.

This system is similar to that of demonstration 28.27 – Roll cylinders down incline, except that with the green ramp, the contact point is at a radius much smaller than that of the outer rims of the yo-yo, as in demonstration 28.33 – Maxwell’s yo-yo, in which the yo-yo is suspended by an axle whose radius is much smaller than that of the yo-yo’s disc. As is true for these systems, we can analyze the yo-yo down incline by considering either its energetics or its dynamics. What is interesting about this demonstration, besides the slow acceleration of the yo-yo as it goes down the green ramp, is that the yo-yo speeds off when it reaches the end of its travel on this ramp.

The energetics tell us that Mgh = (1/2)Mv² + (1/2)Iω². If we take r as the spindle radius, v = ωr, and 2gh = v² + I(v²/Mr²), which gives v² = 2gh/(1 + I/Mr²), and v = √[2ghr²/(r² + I/M)]. Since the yo-yo begins from a standstill, its average velocity is (1/2)v, so the time it takes the yo-yo to travel from the top of the ramp until the rims touch the table is t = 2s/v, or t = 2s√[(r² + I/M)/2ghr²], or t = s√[2(r² + I/M)/ghr²].

Dynamics tell us that the force due to gravity, minus the friction force, which causes the yo-yo to rotate, equals the linear acceleration of the yo-yo, or Mg sin θ - f = Ma. The friction force exerts a torque about the center of the yo-yo, at the radius of the spindle, or fr = Iα. Since α = a/r, we have fr = Ia/r, and f = Ia/r². We thus have g sin θ - Ia/Mr² = a, or g sin θ = a(1 + Ia/Mr²), which gives a = [(g sin θ r²)/(r² + I/M)]. Since sin θ = h/s, we have a = [(ghr²)/s(r² + I/M)]. From the equations of motion, we have v = √(2as), and v = √[(2ghr²)/(r² + I/M)], as we found above. As noted for demonstration 28.27, knowing a allows us to determine the minimum friction necessary to prevent the yo-yo from slipping as it goes down the ramp. We can use either t = √(2s/a) or t = 2s/v to arrive at the same expression we arrived at above for the time it takes the yo-yo to roll down the ramp.

If we treat the yo-yo as a pair of uniform discs, we have I = (1/2)MR², and v = √[(2ghr²)/(r² + (1/2)MR²/M)], which simplifies to v = 2r√[(gh)/(2r² + R²)], and t = (s/r)√[(2r² + R²)/(gh)]. As in the analyses for the other demonstrations mentioned above, we find that the behavior of the yo-yo as it rolls down the ramp does not depend on its mass, but only on how the mass is distributed – its moment of inertia – and on r, the moment friction has about the yo-yo’s axis to exert a torque to cause the yo-yo to rotate. If we insert the dimensions given above, we obtain v = 2(6.32 × 10^-3 m)√[(9.80 m/s²)(0.106 m)/(2(6.32 × 10^-3m)² + (7.62 × 10^-2 m)²] = 0.168 m/s. The time it takes for the yo-yo to come down the ramp is t = 2(0.762 m)/(0.168 m/s) = 9.08 s.

Given our expression for v, we can find the rotational speed of the yo-yo. The relationship between the yo-yo’s linear speed and its rotational speed, while it is rolling down the green ramp, is v = ωr. As soon as the rims touch the table, though, the yo-yo is now rolling on them, and not on the inner spindle. Its rotational speed is the same as before, but now v = ωR. As noted above, r = 6.32 mm, and R = 76.2 mm, so the linear speed of the yo-yo when it touches down on the table is suddenly 12.1 times its original speed, or 2.02 m/s, much faster than it was going just when it touched down. One way of writing all of this is to note that just before the yo-yo touches the table, v = 2√[ghr²/(2r² + R²)], and that when it touches the table, v = 2√[ghR²/(2r² + R²)]. Noting that R = 12.1r, these give v = 2√[gh/147] = 0.165√(gh) just before touchdown, and v = 2√[(145gh)/(147)] = 1.99√(gh) when the rims touch the table. These, of course, equal 0.168 m/s and 2.03 m/s, respectively.

If you place the yo-yo on the red ramp, r now equals R, and v becomes v = 2R√[(gh)/(2R² + R²)] = 2√(ghR²/3R²) = 2√(gh/3) = 1.15√(gh) (= 1.18 m/s). Now when the yo-yo reaches the end of the ramp, R does not change, and the yo-yo continues on at the same speed it attained when it reached the end of the ramp. If you start the yo-yo at the arrow, its starting height is the same as for starting at the top of the green ramp, so it starts with the same potential energy as it has at the top of the green ramp. Its speed when it reaches the end of the red ramp is thus 1.18/0.168 = 7.0 times the speed it attains just before it touches the table when it has rolled the length of the green ramp, but only 1.18/2.03 = 0.58 times the speed it reaches when it touches the table after having rolled down the green ramp. The reason for this is that when the yo-yo rolls on its spindle, significantly more energy goes into rotational acceleration of the yo-yo, and proportionally less energy goes into translation of the yo-yo, than when the yo-yo rolls on its rims.

For the yo-yo just at the end of the green ramp, the translational kinetic energy = (1/2)Mv² = (1/2)(2.870 kg)(0.168 m/s)² = 4.05 × 10^-2 J, and the rotational kinetic energy = (1/2)Iω² = (1/2)[(1/2)(2.870 kg)(7.62 × 10^-2 m)²[(0.168 m/s)/6.32 × 10^-3 m]²] = 2.94 J. The original potential energy Mgh = (2.870 kg)(9.80 m/s²)(0.106 m) = 2.98 J, which, as it should, equals the sum of these two: 4.05 × 10^-2 J + 2.94 J = 2.98 J. Roughly 99 percent of the potential energy has gone into rotation of the yo-yo, and just over 1 percent has gone into translation. Rolled from the same height down the red ramp, the yo-yo acquires translational kinetic energy of (1/2)(2.870 kg)(1.18 m/s)² = 2.00 J, and rotational kinetic energy of (1/2)[(1/2)(2.870 kg)(7.62 × 10^-2 m)²[(1.18 m/s)/7.62 × 10^-2 m]²] = 0.999 J. Thus, when the yo-yo rolls on its rim down a similar incline, about two-thirds of the energy goes into translation, and about one-third goes into rotation. These, again, add up to the total (within rounding error).

References:

1) Resnick, Robert and Halliday, David. Physics, Part One, Third Edition (New York: John Wiley and Sons, 1977), pp. 237, 241, 250-252.