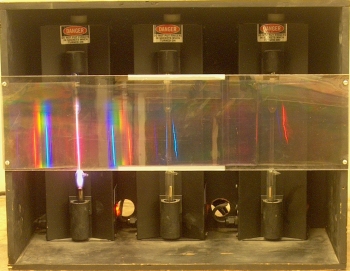
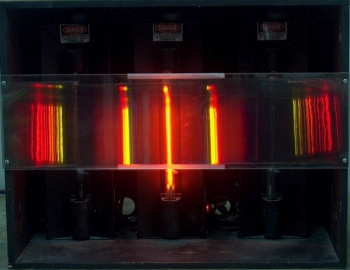
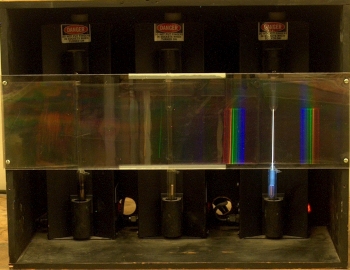
Inside the large box are three power supplies, each with a discharge tube mounted in it. The tube in the one at left contains hydrogen, the one in the middle has a tube that contains neon, and the tube in the one at right contains krypton. Mounted across the front of the box, between layers of plastic, is a transmission grating (750 lines per mm). When you turn one of the power supplies on (each has a switch at bottom right), the light from its discharge tube passes through the grating, and the class sees the various wavelengths of light emitted by the tube, separated according to the angles at which they are diffracted. These emission lines correspond to various electronic transitions of excited atoms relaxing to lower-energy states. It is probably advantageous to darken the room for this demonstration.
This demonstration allows you to show the emission spectra, in the visible region, of any or all of three gases, which are hydrogen, neon and krypton. As noted above, each gas is contained in a discharge tube, which is set in its own power supply. Mounted to the front of the box that contains the three power supplies is a transmission grating whose pitch is 750 lines per mm. With the separation afforded by the grating, the class can see the lines at various wavelengths that compose the emitted light. The photographs below show the light from each discharge tube as seen through the grating. Clicking on each image opens a larger version of it in a new tab or window.
Hydrogen is normally a diatomic molecule, H2. In the electric discharge inside the tube, however, the hydrogen is excited to high enough energy that it dissociates into separate hydrogen atoms. Neon and Krypton are monatomic gases. Thus, the emissions from all of the discharge tubes in this demonstration are from electronically excited atoms relaxing to lower-energy states.
Hydrogen, whose nucleus is a single proton that has a single electron bound to it, is the simplest atom. Niels Bohr proposed a model in which the electron moves about the nucleus in circular orbits. According to classical electromagnetic theory, an accelerating charge radiates electromagnetic energy. This is, in fact, how the antenna of a radio transmitter works. If the electron in a hydrogen atom radiated in this way, we should expect it to lose energy as it did so, and for the wavelength and frequency of the emitted wave to change continuously as well. Since this does not happen, and since excited atoms emit only at certain energies, Bohr proposed that the electron could orbit the nucleus only at certain radii, and that the atom did not emit unless the electron moved from an orbit at one radius to an orbit at a different radius, and then only by emitting discrete quantities of energy. Arnold Sommerfeld expanded Bohr’s model to yield sets of elliptical orbits.
Classically, the force on the electron is
F = Ze2/(4πε0r2), which also equals mv2/r for a circular orbit, or Ze2/(4πε0r2) = mv2/r,
where Z is the number of protons in the nucleus, which for hydrogen equals 1, e is the elementary charge (1.60218 × 10-19 C), ε0 is the permittivity constant (8.85419 × 10-12 F/m (= C2/(N · m2)), and r is the radius of the electron’s orbit about the nucleus. The potential energy of the system is
U = -Ze2/(4πε0r).
(We take the zero of potential energy to be where the electron and the nucleus are at infinite separation; hence the minus sign.) The kinetic energy of the electron is
K = (1/2)mv2, which equals Ze2/(8πε0r).
The total energy, then, is
E = U + K, which equals -Ze2/(8πε0r).
The classical angular momentum associated with the electron is L = mvr. Bohr postulated that the angular momentum was constrained to values that are integer multiples of h/(2π) (= ℏ), or mvr = nℏ. We could continue in SI units, but the equations above and those that follow are easier to handle if we use atomic units. Expressed this way, the force and centripetal acceleration are
Ze2/r2 = mv2/r,
the potential, kinetic and total energy are
U = -Ze2/r, K = Ze2/(2r) and E = -Ze2/(2r).
Combining the equations Ze2/r2 = mv2/r (r = Ze2/(mv2)) and mvr = nℏ (v = nℏ/(mv) gives
r = n2ℏ2/(Zme2).
In these equations, e is in electrostatic units (esu), or statcoulombs (1 statcoulomb = 3.33565 × 10-10 C; 1 C = 2.99792 × 109 statcoulombs; one elementary charge equals 4.80321 × 10-10 statcoulombs), m is in grams, and h = 6.62607 × 10-27 erg·s (so ℏ = 1.05457 × 10-27 erg·s). n is the principal quantum number of the electron. This radius, for n = 1 and Z = 1, if we use the mass of the electron for m (= 9.109384 × 10-28 g) equals 0.52918 Å. This radius, denoted by a0, is called the Bohr radius, and is the definition of the unit called the bohr. (With SI units, the dimensional analysis is straightforward. With atomic units, the constants in the equations above take on different dimensions, so that the expressions above for energy give results that are in units of energy (in this case, ergs), and the expression above for the radius gives a result that is in units of length (in this case, cm, which we have converted to ångstroms).)
In using me to arrive at this value, we have made the assumption that the nucleus does not move; that its mass is so much greater than that of the electron that we may consider it infinite. In reality, the electron and the nucleus move about their center of mass. Though the difference introduced by this is small, we should use the reduced mass, μ = memp/(me + mp). Inserting 9.109384 × 10-28 g for me and 1.672621 × 10-24 g for mp, we obtain μ = 9.104425 × 10-28 g. In the equations below, we will use this value.
If we substitute the expression above for r into the expression for the total energy, we obtain for the various states
E = -Z2e4μ/(2n2ℏ2) or E = -2π2Z2e4μ/(n2h2)
For a transition of an excited atom to a lower-energy state, then,
ΔE = (2π2Z2e4μ/h2)[(1/nf2) - (1/ni2)],
where ni is the principal quantum number of the electron in the initial state, and nf is the principal quantum number of the electron in the final state. This gives the energy difference between the two states, and of the emitted photon, in ergs. Since E = hν and ν = c/λ, if we divide by hc, we obtain the energy in units of inverse wavelength, cm-1:
ΔE = (2π2Z2e4μ/(h3c))[(1/nf2) - (1/ni2)], or ΔE = RZ2[(1/nf2) - (1/ni2)].
where R (= 2π2e4μ/(h3c)) is the Rydberg constant, which for the hydrogen atom is designated RH and equals 109,678 cm-1. (The value we obtain without dividing by hc equals 2.17868 × 10-11 erg, or 2.17868 × 10-18 J.) Balmer was the first to note that the lines emitted by the hydrogen atom in the series that begins in the visible region of the spectrum follow the relation
ν̄ = R[(1/22) - (1/ni2)],
where ν̄ is the wavenumber (1/λ), and R is RH. If the nucleus is considered to have infinite mass, R = 2π2e4me/(h3c), which equals 109,737 cm-1 and is often denoted R∞. For hydrogen-like ions (He+, Li2+, Be3+), R contains the reduced mass of the system and has a different value for each ion, and the general formula is
ν̄ = RZ2[(1/nf2) - (1/ni2)].
The first three lines in the series produced by electrons making the transition from levels with n > 2 to the level n = 2 (in hydrogen) are the red, blue and violet lines we see in the photograph above at left. These have wavelengths of 6,562.8 Å, 4,861.3 Å and 4,340.5 Å, respectively. The fourth line appears at 4,101.7 Å, just within the visible range. This line is difficult to see, but with a bright source and a dark background, some people are able to observe it. This series extends into the ultraviolet, the next four lines appearing at 3,970.1 Å, 3,889.0 Å 3,835.4 Å and 3,797.9 Å. In recognition of Balmer’s work in studying this set of lines, it is known as the Balmer series.
The states between which the electron makes a transition are represented by term symbols. These take the form (2S + 1)LJ, where S is the electron spin, 2S + 1 is the spin multiplicity, L is the total orbital angular momentum of the valence electron(s), represented by S, P, D, F, G, H, etc., for L = 0, 1, 2, 3, 4, 5, etc., respectively, and J, the vector sum of the orbital and spin angular momenta, equals L + S and has the range L + S, L + S - 1, L + S - 2, . . . , |L - S|. These transitions are electric dipole transitions, which means that the two states between which the transition occurs cannot have the same symmetry. (The electric dipole operator is odd, so if the wavefunctions of the two states have the same symmetry, their integral with the operator vanishes.) This means that an electron in a particular orbital (s, p, d, f, etc.) in one level cannot go to the same orbital in a different level; such a transition is said to be symmetry forbidden. (The state to which the electron goes must also have the same spin multiplicity as the initial state. If it does not, the transition is spin forbidden.) In making a transition, the electron must undergo a change in orbital angular momentum of one unit; that is, Δl = ±1. An s electron can go to a p orbital, a p electron can go to either an s orbital or a d orbital, a d electron can go to either a p orbital or an f orbital, etc. In the hydrogen atom, the orbitals associated with each principal quantum number are essentially degenerate; that is, they have the same energy. Thus, the line at 6,562.8 Å is from an electron going from an 2S1/2 state to either a 2P1/2 or a 2P3/2 state (ΔJ = 0, ±1; no transition between two states if both have J = 0), from a 2P1/2 or 2P3/2 state to a 2S1/2 state, from a 2D3/2 state to a 2P1/2 or 2P3/2 state, or from a 2D5/2 state to a 2P3/2 state. In other words, the electron is going either from the 3s orbital to a 2p orbital, from a 3p orbital to the 2s orbital, or from a 3d orbital to a 2p orbital. The other lines in the spectrum arise from similar types of transitions of the electrons from levels with n = 4, 5, 6, etc. down to the level at n = 2.
As is the case with the Balmer series, several other series of hydrogen emission lines are named for those who studied or discovered them.
For transitions from levels with n > 1 to the level with n = 1, the emission lines are in the ultraviolet. The first few of these appear at 1,215.7 Å, 1,025.8 Å, 972.5 Å, 949.8 Å and 937.2 Å. This series is called the Lyman series.
Transitions from levels with n > 3 to the level with n = 3 produce lines in the near infrared. These make up the Paschen series, sometimes called the Ritz-Paschen series. Its first few lines appear at 18,751.1 Å, 12,818.1 Å, 10,938 Å, 10,049.8 Å and 9,546.2 Å.
Further into the infrared, we observe lines produced by transitions from levels with n > 4 to the level with n = 4. This series is the Brackett series. Its first two lines appear at 4.06 μ and 2.63 μ.
The first line of the Pfund series, which arises from transitions from levels with n > 5 to the level with n = 5, appears still further into the infrared, at 7.40 μ.
For the transition between the ground state (n = 1) and the state at which the electron is no longer bound to the nucleus (n = ∞), we have ν̄ = 109,678[(1/12) - (1/∞2)] = 109,678 cm-1. As noted above, had we not divided by hc to obtain the energy in terms of the wavenumber, we would have obtained a value of 2.17868 × 10-11 erg, or 2.17868 × 10-18 J. If we convert this to electron-volts, we have 2.17868 × 10-18 J ÷ 1.602 × 10-19 J/eV = 13.60 eV. This is the ionization potential of the hydrogen atom, that is, the energy it takes to remove the electron from the atom in its ground state.
Neon, with 10 electrons surrounding a nucleus that has 10 protons, and krypton, which has 36 electrons surrounding a nucleus with 36 protons, are far more complex than hydrogen, but their behavior shares certain similarities to that of hydrogen.
Neon
Neon exibits many relatively closely spaced lines in the red, red-orange and yellow regions of the visible spectrum, hence the characteristic orange glow of neon signs (if the fill does not contain other gases, and if the tube wall is not colored) and neon lamps. These lines are close enough together that in this demonstration, their separations are not obvious in the first order spectrum, but we can see them in the second order pattern. The strongest lines in the red appear at 7,245.17 Å, 7,173.94 Å, 7,032.41 Å, 6,929.47 Å, 6,598.95 Å, 6,506.53 Å, 6,402.25 Å, 6,382.99 Å and 6,334.43 Å. Other lines appear at 6,266.50 Å and 6,217.28 Å in the red-orange, at 6,163.59 Å, 6,074.34 Å and 6,030.00 Å in the orange, at 5,944.83 Å, 5,881.90 Å and 5,852.49 Å in the yellow, and at 5,400.56 Å and 5,341.09 Å in the green. All of these lines except for the last one, arise from the transition of an electron from a 3p orbital to the 3s orbital, for a change in valence electron configuration from 2s22p53p to 2s22p53s. Each electron has associated with it four quantum numbers – the principal quantum number, n, the angular momentum (or azimuthal) quantum number, l, the magnetic quantum number, ml, whose values are -l, -l + 1, -l + 2, . . . , l, and the spin quantum number, ms, which can be +1/2 or -1/2. For each of the electron configurations above, there are many ways in which the electrons may be distributed that satisfy the Pauli exclusion principle, according to which no two electrons can have the same values for all four quantum numbers. According to the spin multiplicity and total angular momentum of the electrons, these microstates give rise to various terms. Depending on the value of J, each term may have multiple components, one for each possible value of J (see the discussion on term symbols above). The large number of microstates available in the upper and lower states gives rise to many allowed transitions between the two levels, and thus a large number of lines in the spectrum.
The last line listed above, at 5,341.09 Å, arises from a transition of an electron from a 4d orbital to a 3p orbital, for a change in electron configuration from 2s22p54d to 2s22p53p.
Krypton
As is the case with neon, the levels between which transitions occur comprise many states, and the spectrum exhibits many lines. Some lines that may appear in this demonstration are those at 7,854.82 Å, 7,694.54 Å, 7,601.54 Å, 6,456.29 Å and 6,421.03 Å in the red, orange lines at 6,056.11 Å and 5,993.83 Å, a yellow line at 5,870.92 Å, a green line at 5,570.29 Å, and blue lines at 4,502.35 Å, 4,463.69 Å and 4,453.92 Å. The lines at 7,854.82 Å, 7,694.54 Å, and 7,601.54 Å are due to transitions of an electron from a 5p orbital to the 5s orbital. The lines at 6,456.29 Å, 6,421.03 Å and 6,056.11 Å are due to transitions of an electron from a 6d orbital to a 5p orbital. The lines at 5,993.83 Å, 5,870.92 Å and 5,570.29 Å are due to transitions of an electron from a 5p orbital to the 5s orbital. The lines at 4,502.35 Å, 4,463.69 Å and 4,453.92 Å are due to transitions of an electron from a 6p orbital to the 5s orbital.
References:
1) Herzberg, Gerhard. Atomic Spectra and Atomic Structure (New York: Dover Publications, 1944), 11-28, 37-39, 122, 153.
2) Levine, Ira N. Molecular Spectroscopy (New York: John Wiley and Sons, Inc., 1975), 41-2.
3) Halliday, David and Resnick, Robert. Physics, Part Two, Third Edition (New York: John Wiley and Sons, 1977), pp. 1105-9.
4) Huheey, James E. Inorganic Chemistry (New York: Harper and Row, Publishers, 1978), pp. 810-814 (Appendix on atomic states and term symbols).
5) For the neon spectrum, see Saloman, E.B. and Sansonetti, Craig J. Journal of Chemical Reference Data 33, 1113 (2004), https://physics.nist.gov/PhysRefData/Handbook/Tables/neontable2.htm and associated pages, http://hyperphysics.phy-astr.gsu.edu/hbase/quantum/atspect2.html and http://umop.net/spectra/grotrian.php?elem=Ne&spno=1 .
6) For the krypon spectrum, see Meggers, William F., de Bruin, T. L. and Humphries, C. J. Bureau of Standards Journal of Research 3, 129 (1929), https://physics.nist.gov/PhysRefData/Handbook/Tables/kryptontable2.htm and associated pages, and http://umop.net/spectra/grotrian.php?elem=Kr&spno=1 .