A video of this demonstration is available at this link.
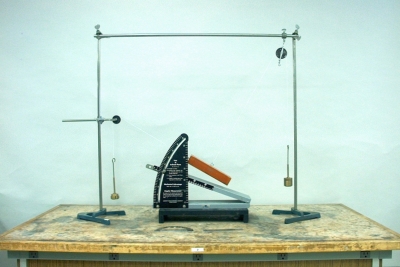
Note: A smaller verion of this demonstration, shown below, is available for use in classrooms outside the Broida lecture hall building.
The red block, which has a mass of 0.979 kg, rests on the incline. Strings from the top face and an end face run over pulleys to mass hangers. If you load the hangers with the proper masses, that is, those masses whose weights balance the two components of the force that gravity exerts on the block when it is resting on the plane, then when you lower the plane, the block remains in place, and the strings remain perpendicular to the block’s surfaces (i.e., the block does not rotate).
Depending on the angle of the incline, it may be more convenient to have the pulley suspended from the hook collar in a different place from that shown in the photograph. Wherever you set it, the collar must be fastened in place before you perform the demonstration. You must adjust the height of the pulley clamped to the rod at right in the photograph, so that the string is parallel to the inclined plane. For angles significantly smaller than 44°, you will also have to move the pulley closer to the rod. Once everything is set, you should place the block on the inclined plane so that the top string is perpendicular to its block face. The small ruler is to check that the end string is parallel to the plane, and the protractor is to check that the top string is perpendicular to the block face.
The two components into which the inclined plane resolves gravity are as shown below:

The dashed line underneath the block shows the original position of the plane (44°). The components of gravity are the dashed vectors below the block, and the resultant is labeled “mg.” The tensions in the strings are equal in magnitude and opposite in direction to the components of gravity, and their resultant (not shown), is equal in magnitude and opposite in direction to “mg.” Since all the masses experience an acceleration of g, we can divide it out of all the vectors and use just the mass of the block and the sine and cosine of the angle of the plane to calculate how much mass we need on each hanger.
The photographs above show the apparatus at its maximum angle, 44°. The smallest angle you can set for this apparatus is 15°. At 44°, the two components are nearly equal, and we have mx = 979 g × sin(44°) = 680 g, and my = 979 g × cos(44°) = 704 g, which are the sums of the masses on the hangers in the photograph. (Even though these are close, their difference is great enough that if you reverse them, the block will not hang at 44°.)
The apparatus shown above uses the large inclined plane and a pair of table clamps. It is possible to make a smaller version with the small inclined plane and a pair of stands:
This setup as shown fits on one of the large grey carts for transportation. Alternatively, it could be carried in pieces to the destination, and then assembled there. Placing the incline on a stand as shown allows you to avoid having the block partially obscured by the vertical plate of the apparatus. It might be possible to increase the range of useful angles by shortening the strings. The block in this version of the demonstration has a mass of 0.406 kg.