A video of this demonstration (with the PASCO unit) is available at this link.
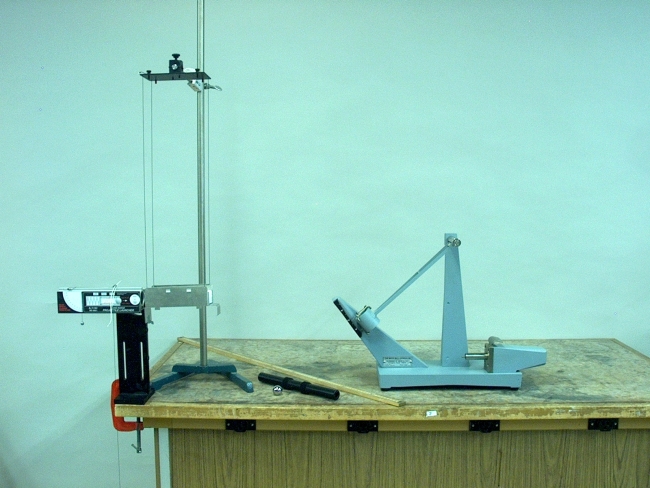
At left in the photograph above is a ballistic pendulum comprising a PASCO launcher and projectile catcher. The launcher fires a 1″-diameter steel ball (66 g) into the catcher (170 g), which swings to the right, to a height that depends on the momentum, and thus the initial velocity, of the ball. The launcher has three speed settings, and a thread trailing from the catcher and held loosely at the front of the launcher, allows you to measure how high the catcher swung after the collision.
At right in the photograph is a Beck ball pendulum (made by Bernard O. Beck and Co.). It fires a 1″-diameter bronze ball (56 g; it is drilled through the center) into the bob of a pendulum (198 g; more details are below). The launcher on this pendulum also has three settings. On the bottom of the catcher is a pawl, which engages a rack towards the end of the pendulum swing, and holds the pendulum at the top of its swing.
The general idea of this demonstration is that when you fire the ball into the catcher, the ball undergoes an inelastic collision with the catcher, which then swings up until all of its kinetic energy is converted to gravitational potential energy, at which point it stops. From the height through which the pendulum has swung, by using the principles of conservation of momentum and conservation of kinetic energy, you can calculate the initial velocity of the ball as it entered the catcher.
While both of these apparatus illustrate the same principles, there is an important difference between them. In the PASCO ballistic pendulum, virtually all the mass of the pendulum is in the ball and catcher; the mass of the thread holding the catcher is negligible. Also, because of the double suspension, the catcher does not rotate as it swings. Thus, the system acts as a simple pendulum, and at the instant of collision, the linear momentum of the ball is completely converted to linear momentum of the catcher and ball as they swing together. Also, the initial kinetic energy of the catcher and ball together is directly converted to gravitational potential energy. The arm of the Beck Ball pendulum, however, is solid, and it is held by a single pivot axle at the top. It is therefore a physical pendulum, and once it begins to swing it has angular momentum. In this pendulum, then, linear momentum is not strictly conserved, but is converted into angular momentum (vide infra).
The PASCO ballistic pendulum:
The PASCO ballistic pendulum is the classic design usually presented in physics books. It has a double suspension, so that, as mentioned above, as the catcher swings, it remains horizontal. Conservation of momentum tells us that the momentum of the ball and catcher together equals the initial momentum of the ball, or mv0 = (m + M)v, where m is the mass of the ball, M is the mass of the catcher, v0 is the initial velocity of the ball, and v is the velocity of the ball and catcher after collision. Conservation of energy tells us that the initial kinetic energy of the ball and catcher equals the gravitational potential energy at the top of their swing, or (m + M)gh = (1/2)(m + M)v2, where m and M are as above, g is the acceleration of gravity, h is the height of the top of the swing above the starting point of the catcher, and v is the velocity of the catcher and ball just after collision. Rearranging the second equation gives v = √(2gh). substituting this into the first equation gives v0 = ((m + M)/m) √(2gh). As noted above, the mass of the ball is 66 g, and the mass of the catcher is 170 g, for a total of 236 g. g, of course, is 9.8 m/s (or 980 cm/s). Bringing out √(2g) and inserting m, M and g gives v0 = 158.3√h cm/s.
Because the collision between the ball and catcher is inelastic, kinetic energy is not conserved in the collision. The initial kinetic energy of the ball is (1/2)mv02. The kinetic energy of the ball and catcher, as noted above, is (1/2)(m + M)v2. Dividing the kinetic energy after collision by the initial kinetic energy of the ball, with the substitution v = √2gh, we get [(1/2)(m + M)(2gh)]/[(1/2)m((m + M)/m)22gh], or K.E.after/K.E.before = m/(m + M). For our PASCO system, this equals (66 g)/(66 g + 170 g) = 0.28, or 28%. The lost kinetic energy has gone into deformation of the inside of the catcher, and heat. We can see from this ratio that the greater the mass difference between the ball and catcher, the less the kinetic energy that remains after the collision (so the more that ends up as deformation and heat).
To operate the launcher, insert the ball into the muzzle, then use the black, cylindrical pusher to push the ball further into the muzzle, until the piston inside reaches the desired detent. There are three detents, marked “Short Range,” “Medium Range” and “Long Range.” A yellow bar indicates to which detent you have inserted the ball. To release the launcher, pull up on the string connected to the lever on top of the launcher barrel. After the pendulum has swung, by holding the trailing string at the gun so that the length pulled out from the front of the gun cannot change, you can swing the catcher up until the string is taut, and measure its height with the meter stick. (The demonstration is now set out with a stand for the meter stick, so that you can set the meter stick near where the cradle was at its maximum height, and have both hands free to manipulate the string and cradle. Alternatively, you could ask a student to assist you.)
The Beck ball pendulum:
As noted above, in the Beck ball pendulum the ball catcher is part of a rigid arm, which swings about a pivot on which it is mounted at its top end. This arm thus behaves as a physical pendulum, and the initial linear momentum of the ball is converted into angular momentum of the ball plus pendulum arm. The resulting rotational kinetic energy is then converted into gravitational potential energy as the arm swings upwards. We will see that the equations above should overestimate v0 somewhat, but that the rather significant friction in the pawl and rack mechanism mostly cancels this error.
At the instant in which the ball, whose linear momentum is mv, collides with the pendulum, it has angular momentum with respect to the pendulum pivot. Conservation of angular momentum gives mLv = Iω, where m is the mass of the ball (56.4 g), L is the distance from the center of the pivot to the center of the pendulum bob (30.2 cm), v is the intial velocity of the ball, I is the moment of inertia of the pendulum, and ω is the angular velocity of the pendulum. Conservation of energy gives us (1/2)Iω2 = Mgh, where M is the mass of the pendulum with the ball (254.1 g), g is, of course, the acceleration of gravity, and h is the change in height of the center of mass of the pendulum (with ball) from the bottom to the top of its swing. A small dot just above the ball catcher is supposed to indicate the center of mass of the pendulum with ball. Balancing the arm on a steel ruler shows the center of mass to be somewhat higher. A second, somewhat larger, red dot shows where the measured center of mass is. For a physical pendulum, the period, T, equals 2π√(I/MgR), where R is the distance from the pivot to the center of mass of the pendulum (27.0 cm for this one). Timing the swing of the pendulum (with ball) gives a period of about 1.08 s. Rearranging this gives I = (T2MgR/4π2). Rearranging our equation for conservation of energy gives ω = √(2Mgh/I). Substituting this into our equation for conservation of momentum, then simplifying, gives v = (MgT/πmL)√(Rh/2). Bringing √h out and inserting all the values gives v = [(254.1 g)(980 cm/s2(1.08s)/(3.1416)(56.4 g)(30.2 cm)]√[(27.0 cm)/2]√h, or v = 184.7√h cm/s.
The analysis above for the PASCO apparatus gives for the Beck ball pendulum v = (M/m)√(2gh), or 199.4√h cm/s, which is about 8% high.
Of course, the note above regarding loss of kinetic energy in the collision of the ball and pendulum holds. By the analysis above, the ratio of kinetic energy after and before is K.E.after/K.E.before = [(1/2)Iω2]/[(1/2)mv2] = [(1/2)I(2Mgh/I)]/[(1/2)m(M2g2T2/π2m2L2)(Rh/2)] = (4π2mL2)/(MgT2R). We can also write this as (m/M)(L2/R)(4π2/gT2). (The first term of this expression is the ratio from the analysis for the PASCO pendulum, (m/(m + M)).) For our system, this equals (56.4 g/254.1 g)(30.22 cm2/27.0 cm)(4π2/(980 cm/s21.082s2)) = (0.222)(33.8)(0.0345) = 0.26, or 26%, similar to that for the PASCO apparatus. The lost kinetic energy has gone into spreading the claws in the ball catcher, and into heat.
To cock the gun, place the ball on the rod and push it towards the gun. When you reach the first detent, the trigger plate will spring up and catch the gun ram. To go to the second detent, while pushing the ball, press down on the trigger plate. As soon as the ball moves further, release the plate, and keep moving the ball. When you reach the second detent, the trigger plate will spring up and catch the ram again. To reach the third detent, repeat this process, or, while holding down the trigger plate, push the ball all the way in, then release the trigger plate and allow the ball to come back until the trigger plate catches in the detent.
With the gun at the first detent, the pawl typically comes to rest on the first or second tooth of the rack (number 5 or 6 on the scale). At the second setting, the pawl catches the fifth or sixth tooth on the rack (number 10 or 11 on the scale). With the gun set at the third detent, the pawl reaches the tenth or eleventh tooth on the rack (number 15 or 16 on the scale).
The table below shows for each gun setting the ball velocity as measured by its projectile motion (that is, firing the ball horizontally and measuring at what distance it hits the floor; average of three trials), the height of the pendulum swing, h, the ball velocity as calculated by the analysis for the physical pendulum, vpend, and the ball velocity as calculated by conservation of linear momentum, vlin. The Δ% columns are percent differences relative to the velocity measured by projectile motion.
Gun setting 1 Gun setting 2 Gun setting 3 We can see that the results of the linear analysis are fairly close to the projectile measurements, and that the values from the rotational analysis are all somewhat low. As noted above, the pawl and rack mechanism introduces significant friction towards the end of the pendulum’s travel. This slows the pendulum and reduces h somewhat. This, of course, affects the results for both analyses as indicated in the table. We should expect that without the friction from the rack, the rotational analysis would yield values close to those given by the projectile measurement, and the linear analysis would yield values that are about 8% high.
Several papers contain interesting analyses of the Beck ball pendulum. They are listed below, and are accessible from any computer connected to the UCSB network.
References:
1) Resnick, Robert and Halliday, David. Physics, Part One, Third Edition (New York: John Wiley and Sons, 1977), pp. 194-5, 233-5, 310-11, 313-15.
2) Sears, Francis Weston and Zemansky, Mark W. College Physics, Third Edition (Reading, Massachusetts: Addison-Wesley Publishing Company, Inc., 1960), pp. 165-6.
3) Wall, C.N. American Journal of Physics, 36(12), 1161-3 (1968) (Review of the Beck ball pendulum).
4) Sandin, T.R. American Journal of Physics, 41(3), 426-427 (1973) (Nonconservation of Linear Momentum in Ballistic Pendulums).
5) Sachs, A. American Journal of Physics, 44(2), 182-3 (1976) (Blackwood pendulum experiment revisited).
6) Wicher, Enos. American Journal of Physics, 45(7), 681-2 (1977) (Ballistics pendulum).
7) Instruction Manual for EB-03 Beck Ball Pendulum (accessible from any computer).