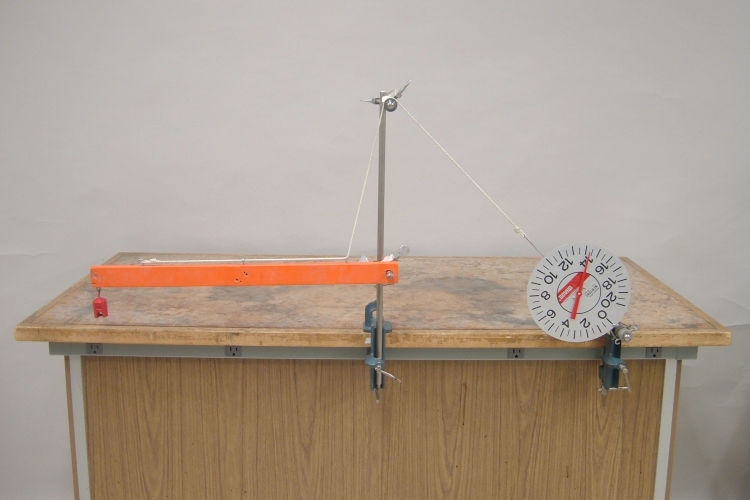
This apparatus mimics the action of the biceps, the main muscle in the upper arm responsible for raising the forearm and hand. It shows how much greater the tension must be in the biceps, than the weight exerted by the mass at the end of the forearm, in order to support that weight. With the system unloaded, the scale reads about 7.5 N. In order to balance the 0.98-N force exerted by the 100-g mass hanging at the end, the scale must provide roughly an additional 7 N of tension. The eyes near the pivot and near the center of the orange board, are split so that you can slip the rope out. When you do this (while leaving the mass hanging at the end), you decrease the tension in the rope. (A plastic pulley on ball bearings, at the top of the apparatus, essentially eliminates rope drag over the rod, so the apparatus works smoothly.)

This apparatus is a model of the upper arm and forearm, in which the orange board represents the forearm, its pivot represents the elbow, and the rod at the top represents the shoulder. The section of rope between the board and the rod at the top represents the biceps muscle, and the scale provides the tension that the biceps would produce. The biceps brachii gets its name from the fact that near the top, the muscle splits into two parts, which originate from two points on the shoulder blade (scapula). Hence, two heads, or biceps. The distal end inserts into the radius near the elbow, as does the rope into the orange board at the eye nearest the pivot. (See, for example, https://en.wikipedia.org/wiki/Biceps and https://www.registerednursern.com/biceps-brachii/.)

The hand, forearm, elbow and biceps together comprise a “class three” lever. This is a lever for which the “load” is at one end, the fulcrum is at the other end, and the “effort” is between the load and the fulcrum. The hand holding a mass, whose weight constitutes the load, is represented by the 100-g mass at the end of the orange board. If we ignore the weight of the forearm itself, and that of the orange board, the torque exerted about the elbow, or the pivot rod at the opposite end of the board, is τ_hand = r_hand × F_hand, where r_hand is the position vector from the pivot to where the mass hangs, and F_hand is the weight exerted by the mass, mg, or about 0.98 N. The magnitude of this torque is rmg sin θ, where θ is the angle between r_hand and F_hand. If θ equals 90 degrees, then sin θ equals 1, and τ = rmg. Torque is often called the moment of force, and r is the moment arm. If r_hand and F_hand are not perpendicular, then the moment arm is r sin θ, the perpendicular distance from the pivot to where the mass hangs.

As noted above, the spring scale takes the place of the biceps muscle, and is attached to the board near the pivot as the biceps is attached to the radius near the elbow. The scale exerts a torque that opposes that exerted by the hanging mass. This torque is τ_biceps = r_biceps × F_biceps, where the subscript refers to the rope attached to the spring scale, and F_biceps is the tension in the rope. The same caveat regarding the effect of the angle between r and F on the magnitude of the moment arm and thus of the torque, of course, applies here as did for the torque exerted by the hanging mass.

The distance between the pivot and the hanging mass is 58.0 cm, and the eyes are placed at distances of 8.5 cm, 28.5 cm and 49.0 cm from the pivot. The mass is thus 58.0/8.5 = 6.8 times as far from the pivot as the point at which the spring scale exerts its torque. The spring scale, which is providing the effort, thus has a mechanical advantage of 1:6.8, or about 0.15:1, which is actually a mechanical disadvantage. When the mass is not hanging from the end of the orange board, the spring scale reads approximately 7.5 N. As the photograph above shows, with the mass hanging from the end, the scale reads slightly less than 14.5 N. In order to balance the 0.98-N force exerted by the hanging mass, then, the scale must increase the tension by about 7 N. With the orange board in the position shown, the tension applied by the rope is nearly perpendicular to its line to the pivot, which is slightly longer than the horizontal distance between the pivot and where the tension is applied. There is a similar small offset for the hanging mass. Despite these slight offsets, the behavior of the model is fairly close to that described by the equation above with the nominal distances above and sin θ ≈ 1. You can change the mechanical advantage/disadvantage by slipping the rope out of the first, and then the second, eye from the pivot. When you do this, you increase r_biceps, and thus increase the mechanical advantage and reduce the tension in the rope. r_biceps and F_biceps get farther from being perpendicular, so the increase in r_biceps is less than that in r, but it is still enough to increase the mechanical advantage and reduce the tension.

As noted above, the spring scale has a mechanical disadvantage (mechanical advantage less than one) relative to the load. This is also true of the biceps muscle relative to the hand. Just as the spring scale, in order to support the 100-g mass, must provide tension that equals several times its weight, so must the biceps muscle provide tension that is several times greater than the weight of an object, in order to lift it with the hand. When you remove and replace the 100-g mass at the end of the orange board, however, that end of the board moves about seven times the distance that the eye, where the rope is attached near the pivot, moves. The hook of the spring scale, of course, also moves through this shorter distance. Similarly, when the biceps contracts, it raises the hand over a much longer distance than that over which it contracts. Also, the speed with which the hand moves is greater than the speed at which the biceps contracts, in the same ratio as the distances over which they move. This conversion of a small motion where the effort is applied, to a large motion where the load is, is a useful property of levers for which the mechanical advantage for the effort relative to the load is significantly less than one. To achieve a broad range of motion with moderate muscle contraction, many (most?) of the joints in the body are designed as levers for which the mechanical advantage for the muscle (the effort) is significantly less than one, as is the biceps-elbow-forearm system illustrated by the model in this demonstration.

References:

1) Resnick, Robert and Halliday, David. Physics, Part One, Third Edition (New York: John Wiley and Sons, 1977), pp. 231-233.