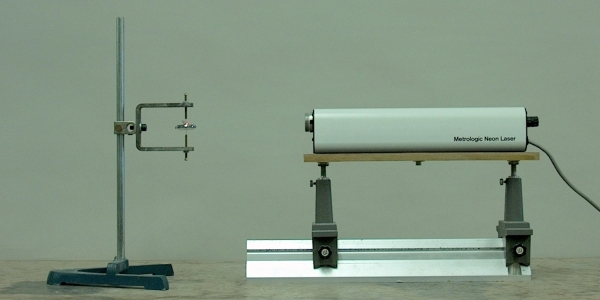
Held between pivot points in the yoke at left in the photo above is a spring-driven wristwatch. This type of watch keeps time by means of an escapement that is governed by a balance wheel and hairspring. When the cog at the end of the watch’s gear train engages one of the pins (a jewel or other rectangular process in a more expensive watch mechanism) on the escapement lever, the opposite end of the lever spins the balance wheel. On its back spin, the balance wheel flips the lever in the opposite direction, engaging the opposite pin (or jewel) with the cog, and the cycle repeats. A mirror mounted on the back of the watch allows you to reflect a laser beam onto a wall in the lecture hall. The oscillatory motion of the watch causes the laser spot on the wall to move back and forth along a line (vide infra).

The frequency at which the balance wheel oscillates depends on its moment of inertia and the force constant of the hairspring, and is:

where k is the spring constant and I is the moment of inertia of the balance wheel. This is an example of simple harmonic motion, but with torsion instead of linear motion.

Since the balance wheel rotates, it has angular momentum (L=Iω, where ω is the balance wheel’s angular velocity), and the sense of its angular momentum vector alternates between opposite directions along the axis of the balance wheel as the balance wheel oscillates back and forth. Unless there is some external torque acting on the watch, its angular momentum must remain constant (zero), that is, it must be conserved. The balance wheel, when it rotates, thus imparts an opposite angular momentum to the rest of the watch, causing it to rotate in the opposite direction. Since the watch case and works are together considerably more massive than the balance wheel, the motion is slight, and in normal handling and wearing of such a watch, one does not notice this. If, however, the watch is placed so that it is free to pivot about an axis perpendicular to its face, one can see the case oscillate back and forth. In the apparatus shown above, the watch has a small mirror glued to its back, with a small tilt upwards. As the case oscillates the mirror rotates with it through a small angle, so that if you reflect a laser beam off the mirror and project it onto a wall, the laser spot will sweep along a line on the wall. Below are an expanded view of the apparatus and a long exposure of the projected spot on the wall:

When the apparatus is placed so that the mirror on the watch is approximately six meters from a wall, the distance from the mirror to the projected spot is about 6.4 meters, and the path along which the spot travels is about 18 centimeters long. For reference, the square in the photograph at right is approximately 30 centimeters across. If you place the apparatus at one side of the lecture hall and project the beam across to the wall at the opposite side, the distance over which the spot travels will be considerably longer than that shown above.

You can use this demonstration to show the conservation of angular momentum and perhaps also to illustrate simple harmonic motion, or at least in conjunction with other simple harmonic motion demonstrations.