A video of this demonstration is available at this link.
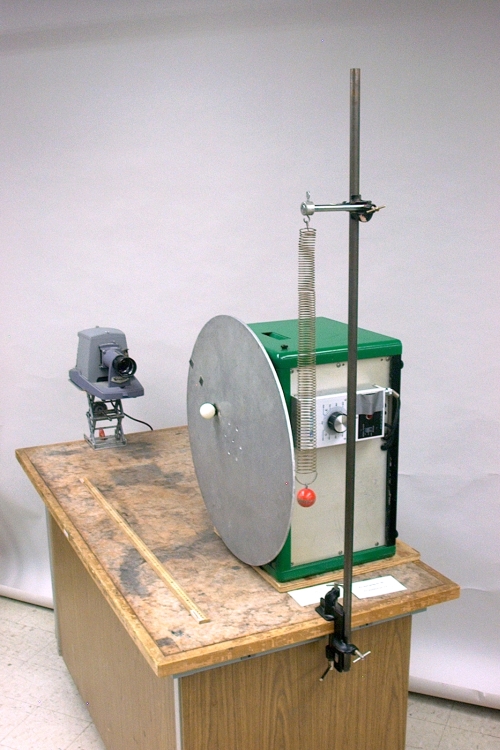
A smaller apparatus that illustrates the relationship between linear and circular motion, shown below, is available for use in classrooms outside the Broida lecture hall building.

A video of this demonstration is available at this link.
A smaller apparatus that illustrates the relationship between linear and circular motion, shown below, is available for use in classrooms outside the Broida lecture hall building.
Turn on the projector. Then turn on the turntable, on the “HI” setting. (This setting is the second click of the switch. This is important! The frequency of oscillation of the mass-spring is about 55 cycles per minute, or about 0.92 Hz, which corresponds to a setting of about 80 on the dial. This should be set before class, and should not be changed.) Now pull the red ball down so that its shadow lines up with that of the orbiting ping-pong ball when it is at its lowest point. At the appropriate time (just as the ping-pong ball is at this turning point), release the red ball. The two shadows should then overlap as the mass-spring oscillates and the ping-pong ball goes around its circle.
When an object travels in a circle at constant speed, if we take the center of the circle as the origin of a pair of coordinate axes, then the x and y coordinates of the object both vary sinusoidally. Thus, the projection of the object’s motion along either axis (or any line parallel to a tangent of the circle) is a sinusoidal variation of position along a straight line. If we illuminate the object in a direction that is parallel to the plane in which it moves, then the moving shadow cast by the object is such a projection, and its position varies sinusoidally. We can then set a linear oscillator parallel to the tangent of the circle that is perpendicular to the direction of the illumination, and line it up so that the range over which its shadow travels corresponds to that for the object moving in a circle. If we adjust the period of the object’s circular motion to correspond with the period of oscillation of the linear oscillator, and then set the linear oscillator in motion at the proper time, the shadows of the object moving in a circle, and of the linear oscillator overlap. A vertical mass-spring oscillator is such a system; the mass oscillates along a straight line, and its displacement from the equilibrium position varies sinusoidally. The motion of the mass on the spring is thus similar to the linear projection of the motion of the orbiting ping-pong ball, and we can make the projected shadows of the moving ping-pong ball and oscillating mass overlap.
This demonstration provides a good way to illustrate the concept of phase for a linear sinusoidal oscillator. One complete cycle of motion of the mass-spring corresponds to one complete orbit of the ping-pong ball, or an angular displacement of 2π radians or 360 degrees. One half cycle is then π radians or 180 degrees, one quarter cycle is π/2 radians or 90 degrees, etc. (See also demonstration 40.18 -- Circular vs. pendular motion.)
For classes that meet in locations outside Broida Hall, the apparatus shown below is available.
It is probably possible to use this device under a document camera, set on a white background, but an overhead projector may work better. A projector is available, as is a cart to transport it. The photograph below shows how the image from an overhead projector looks.