A video of this demonstration is available at this link.
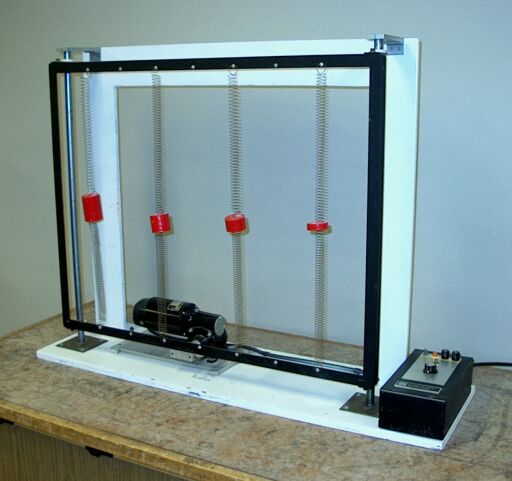
Four different masses (red) are attached at top and bottom, by identical springs, to a rectangular frame (black). This system thus comprises four mass-spring oscillators, all having the same spring constant, but each with a different mass from the others, and so each with a different natural frequency of oscillation. A crank driven by a variable-speed motor sets the frame oscillating up and down. When you set the frame oscillating, unless the frequency matches the natural oscillating frequency of one of the mass-spring oscillators, all of the masses move very little. As you adjust the motor speed, when the frequency at which the frame oscillates matches the natural frequency of one of the mass-springs, the motion of its mass becomes greater and greater, until it oscillates wildly. If you start at a frequency that is lower than that of the lowest-frequency oscillator (the one with the greatest mass, at left) and then slowly raise it, as you reach the natural frequency of each oscillator in turn, you set it oscillating wildly.
The mass-spring oscillators in this apparatus are similar to that in demonstration 40.45 -- Driven mass-spring. The only difference is that, whereas the mass in the driven mass-spring hangs from the end of a single spring, the masses in the apparatus shown above are suspended between two springs. When one of the masses is displaced upward from its equilibrium position, this compresses the top spring and extends the bottom spring by the same distance. When the mass moves downward, it compresses the bottom spring and extends the top spring by the same distance. Because the mass extends and compresses the two springs by the same distance, their spring constants add linearly; the tensive force due to the extended spring increases by the same amount as does the compressive force due to the compressed spring. (When both springs are tension springs, the increase in force due to the extended spring is the same as the decrease in force due to the retracted spring.) Hence, for the mass suspended between two springs, we may use the same equation as for the driven mass-spring in demonstration 40.45, which, if we include damping, is:
m(d2x)/(dt2) + c(dx/dt) + kx = F0 cos ωt,
where F0 cos ωt = FD(t), the periodic driving force. If we divide through by m, define the damping factor γ = c/2m, and note that the natural frequency of the mass-spring oscillator is ω0 = √(k/m), this yields the solution
x = Ae-γt sin (ω′t + φ) + ((F0/m)/[(ω02 - ω2) + 4γ2ω2]1/2) cos (ωt - δ).
The first term on the right, which describes the motion of a damped, free oscillator, decays over time, and is thus called the transient part of the solution. The amplitude factor A equals (x0 - C cos δ)/sin φ, and ω′ = √(ω02 - γ2). The second term on the right does not decay with time, and is called the steady-state part of the solution. We can see that if the damping is large, the amplitude of oscillation is small. Conversely, if the damping is small, the amplitude can be large, depending on the other factors in the equation. We also see that if the frequency of the driving force is much different from the natural oscillating frequency of the mass-spring, the amplitude of the motion is small, and that as the driving frequency approaches the natural oscillating frequency, the amplitude increases. The phase factor δ gives the relationship – the lag – between the driving force and the motion of the mass-spring, and it equals tan-1 [2γω/(ω02 - ω2)].
For the lowest-frequency mass-spring, you may be able to see this in the motion of its mass relative to that of the frame. If the driving frequency is much lower than the natural frequency of oscillation of the mass-spring, the motion of the mass is almost in phase with that of the frame (δ ≈ 0). As ω increases, the phase angle, and thus the lag between the motion of the mass and that of the suspension point, slowly increases. (Since δ is subtracted from ωt, it is a negative phase angle.) As ω approaches ω0, the phase angle increases more and more quickly. When ω = ω0, δ = 90°; the motion of the mass lags that of the suspension point by one quarter cycle. As ω increases further, δ continues to increase. When ω is no longer close to ω0, δ inreases more and more slowly. When ω greatly exceeds ω0, δ approaches 180°.
If we plot the amplitude of the steady-state term as a function of the driving frequency, the curve we obtain is low at points far from ω0 on either side, and rises from either side as ω approaches ω0, to reach a maximum at ω0. The breadth of the peak – its full width at half maximum – at ω0 depends on the damping, γ. For small γ, this width equals γ. Its full width at half maximum is Q = ω0/γ. (This quantity is often called the quality.) Thus, as you move the dial toward the setting that corresponds to the natural frequency of the mass-spring, the motion becomes greater and greater.
For this particular system, damping is very small, and the (ω02 - ω2) in the denominator of the amplitude factor of the steady-state term dominates. Thus, the amplitude can grow very large when resonance occurs.
References:
1) https://scienceworld.wolfram.com/physics/SpringsTwoSpringsandaMass.html.
2) Braun, Martin. Differential Equations and Their Applications (New York: Springer-Verlag, 1978) pp. 161-164.
2) Feynman, Richard P., Leighton, Robert B. and Sands, Matthew. The Feynman Lectures on Physics, Volume I (Reading, Massachusetts: Addison-Wesley Publishing Company, 1963) pp. 23-3 to 23-5.
3) Resnick, Robert and Halliday, David. Physics, Part One, Third Edition (New York: John Wiley and Sons, 1977), pp. 323-325.
4) http://hyperphysics.phy-astr.gsu.edu/hbase/oscdr.html#c1 and sections and links below.
5) http://hyperphysics.phy-astr.gsu.edu/hbase/oscdr2.html#c1 and links therein/sections below.