A video of this demonstration is available at this link.

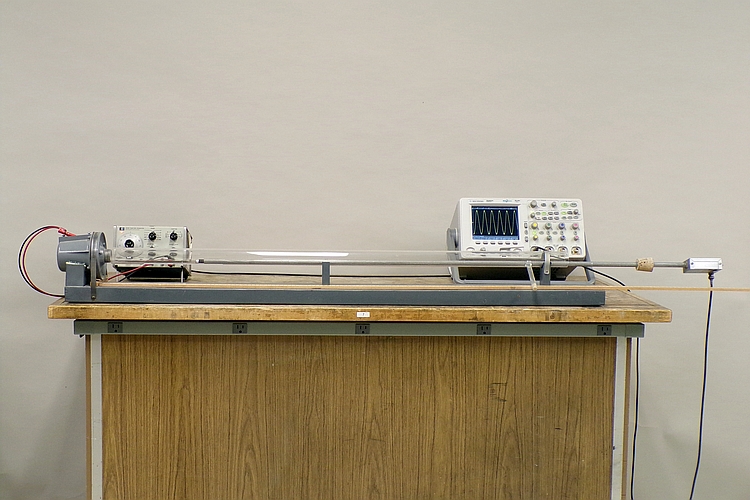
A signal generator feeds a sine wave to a loudspeaker driver that sits at one end of a glass tube that is open at both ends. In synchrony with the sine wave, the signal generator provides a trigger to the oscilloscope. A microphone on the end of a long stalk allows you to probe inside the glass tube, anywhere along its length. The output of the microphone is connected to one of the oscilloscope inputs. When you place the microphone inside the tube, you can see the sine wave on the oscilloscope. If you tune the frequency of the sine wave to that of a harmonic of the tube, then as you move the microphone along the length of the tube, the variation in the amplitude of the sine wave shows the pattern of the standing wave inside the tube.

When you send a wave through a particular medium, if that medium has well-defined ends, as a vibrating string or an air column contained within a tube, when the wave reaches the opposite end of the medium, it is reflected back on itself. Whether it is reflected in phase or out of phase with itself depends on whether that particular end of the medium is “closed” or “open.” (See demonstration 40.66 -- Longitudinal wave machine with free or fixed end. This phenomenon is perhaps easier to see with demonstration 40.60 -- Transverse wave machine with free or fixed end.) With a tube, at an open end, the air is not axially constrained by the tube, so the motion of the air is greatest there, and there is an antinode (the reflection is in phase). For the glass tube in this demonstration, both ends are open, so there is an antinode at each end. If the frequency of oscillation is such that the length of the tube corresponds to an integral number of half wavelengths, then the superposition of the wave with its reflections at the ends of the tube results in a pattern of alternating antinodes and nodes, with an antinode at each end of the tube, and the number of nodes and antinodes between them depending on how many half wavelengths fit within the length of the tube. Such a pattern is called a standing wave. The first such pattern, that is, the one corresponding to the lowest frequency that produces a standing wave, has one antinode at each end of the tube, and one node at the middle of the tube. This frequency is called the fundamental, or the first harmonic. If we call the fundamental f, we should observe harmonics at f, 2f, 3f, 4f, 5f, etc. Each harmonic above the fundamental has one more node and one more antinode than the one below it. For example, the second harmonic has one antinode at each end of the tube, one antinode in the middle, and two antinodes, each centered between the middle and one end of the tube. The third harmonic has four antinodes and three nodes, etc.

It is important to note, especially for the purposes of this demonstration, that the nodes and antinodes to which the text above refers, are displacement nodes and antinodes. They refer, respectively, to regions in which the motion of the air molecules is least, and those in which it is greatest. A microphone, however, responds to sound pressure. Where the air is not constrained, and the motion is greatest, the pressure is least. Where the air is constrained, and the motion is least, the pressure is greatest. Hence, displacement nodes correspond to pressure antinodes, and displacement antinodes correspond to pressure nodes. Thus, when you use the microphone to probe the standing wave of the first harmonic in the glass tube, the amplitude you see at the open ends is very small, and it is maximum at the middle of the tube. The second harmonic shows minimum amplitude at the ends and middle of the tube, with two maxima between the ends and the middle, etc.

The length of the tube is about 1.14 meters, which means that the fundamental has a wavelength of about 2.28 meters. If we assume that the speed of sound at room temperature is about 344 m/s (see demonstration 44.03 -- Speed of sound in air vs. helium), we should expect to observe the fundamental for the tube at a frequency of about 150 Hz, and higher harmonics at integral multiples of 150 Hz.

To find each harmonic, start with the frequency of the signal generator set somewhat below 150 Hz, and the volume moderately low. Slowly increase the frequency. As you get close to the fundamental, the intensity of the sound will increase. Keep increasing the frequency until you have gone past the point where the sound is loudest, and then go back to it. When you now probe the inside of the tube with the microphone, you should see the oscilloscope trace go from a small amplitude at the end of the tube, up to a maximum at the middle, and back down to a small amplitude at the other end. Now increase the frequency to somewhat below twice that of the fundamental, and slowly increase it as you did to find the fundamental, listening for the increase in sound intensity. Adjust the volume on the signal generator as necessary. The frequencies you find for the various harmonics should be fairly close to multiples of 150 Hz. You can easily go from the fundamental up three octaves, to the eighth harmonic at about 1,200 Hz.

If you wish, you can use the cork stopper that is on the microphone stalk (near the box at the right end of the stalk, in the photograph above) to close one end of the tube. When you do this, the wave at the closed end undergoes a change of sign upon reflection, so the only standing waves that can form are those that have a displacement antinode (pressure node) at the open end and a displacement node (pressure antinode) at the closed end. Thus, the harmonics you obtain are those for which the length of the tube equals odd multiples of quarter wavelengths – λ/4, 3λ/4, 5λ/4, etc. The fundamental has a wavelength of about 4.56 meters, which divided into 344 m/s gives a frequency of about 75 Hz. You can clearly hear this resonance, but because the speaker does not respond well at this frequency, the sinusoid has rather intense noise riding on it. You can, however, use the cursors on the oscilloscope to measure the period of a cycle. Such a measurement gives a frequency for the fundamental of about 76 Hz. We expect to find harmonics above this at spacings of about 150 Hz. That is, if we take the frequency of the fundamental as 75 Hz, we should expect harmonics at 225 Hz, 375 Hz, 525 Hz, etc. The frequencies at which you find the odd-quarter-wavelength harmonics should be fairly close to these. (The waveforms and standing wave patterns for all the other harmonics are much clearer than for the fundamental.)