Chladni plates

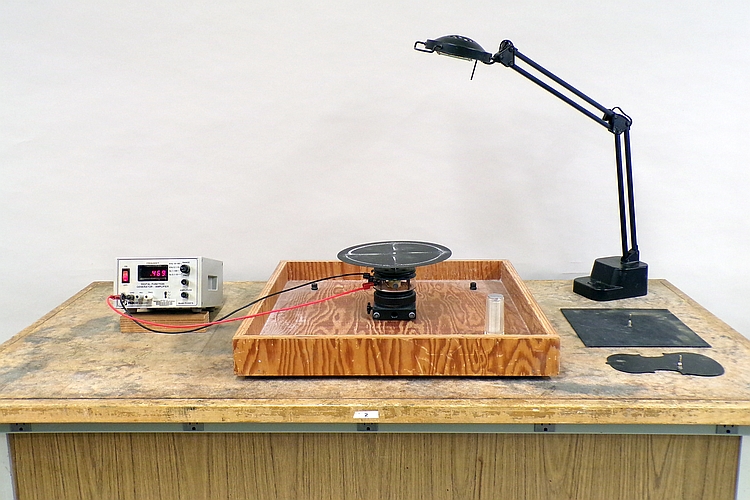
A signal generator feeds a variable frequency mechanical drive, shown above with a circular plate attached. When you drive the plate at certain frequencies, you produce standing wave patterns, which you can make visible by sprinkling sand on the plate. Also available are a square plate and a plate in the shape of a violin. The lamp is there in case it is necessary for obtaining a good image to project to the class.

Several demonstrations, most notably 44.75 -- Standing waves on cord, 44.15 -- Weighted string sound box, 44.18 -- Sonometer, and 44.21 -- Guitar, show vibrations and standing waves in a one-dimensional medium, a string, and demonstration 44.24 -- Idiophones, shows vibrations in bars, in which the dominant motion is along one axis. This demonstration allows you to show the standing wave patterns that occur in a metal plate, in which waves can travel in various directions along a plane. When you cause a plate to vibrate at a frequency at which a standing wave pattern forms, you can make the pattern visible by sprinkling sand on the plate. The sand moves away from the antinodes and settles in the nodes, revealing the pattern. Plates driven in this manner are called Chladni plates, and the standing wave patterns are called Chladni figures, after Ernst Chladni, who studied such patterns extensively. You can read about him and his work in a Wikipedia entry here, and in an item that appeared in APS News here.

When Chladni performed his experiments, he mounted the plates at the center, and he drew a violin bow over the edges at various places to set them vibrating. In this demonstration, we use a function generator connected to a variable frequency mechanical drive, with the center of the plate mounted on the drive. This drive is virtually identical to the working mechanism of a loudspeaker (see demonstration 68.54 -- Loudspeaker). It consists of a voice coil that sits between the pole faces of a circular magnet and is attached to a central core that is held by a dual suspension. This has at the top a rod whose end has a socket that accepts a banana plug. Each plate has attached to it a banana plug, which you insert into the rod. Before mounting or removing a plate, make sure that the lock is engaged in the groove in the rod. Otherwise, pulling up or pressing down on the banana plug to insert or remove it, can distort the suspension of the drive. Once the plate is mounted, release the lock to perform the demonstration. Use only as much sand as necessary to show the nodal lines. For some of the modes that occur at high frequencies, if there is too much sand, the additional mass makes it difficult to excite them to obtain the standing wave pattern.

Available are a circular plate, 24 cm in diameter (~0.86 mm thick), a square plate, 24 cm on each side (also ~0.86 mm thick) and a plate in the shape of a violin (~0.76 mm thick). The violin-shaped plate is a 56%-scale copy of a violin built in London by Emanuel Whitmarsh (so it is slightly larger than half the size of a normal violin body, and similar in shape). Both the circular plate and the square plate have the banana plug attached at the center. The violin-shaped plate is not mounted in the center, but at the point where the sound post is supposed to be. The sound post is a dowel set between the top (or top plate) and back of the violin to provide a node for the waves produced in the top by the rocking motion of the bridge caused by the vibrating strings. (All members of the violin family have a sound post.) This determines the frequencies and intensities of the various harmonics that resonate in the instrument, and the nature of the standing wave patterns they form. The exact placement of the sound post can thus have a profound effect on the sound quality of the instrument. In a violin, the top is fixed to the side (or rib), which produces a node a small distance from the edge of the plate all around it. The edge of the violin-shaped Chladni plate is free, and whereas the top of a violin is curved, the Chladni plate is flat. Despite these differences, it is still interesting to observe the standing wave patterns produced in the violin-shaped Chladni plate.

Depending on the shape of the plate, the geometry, or symmetry, of the waves that form can vary. For example, a circular plate might oscillate about radial nodes, to vibrate in halves, quadrants or other sections, or it might oscillate about circular nodes. In addition, some of these modes might combine, so that the plate simultaneously undergoes different types of motion. The patterns shown below should illustrate this. It bears noting that the point where the plate is fixed to the driver is a node, since the motion is constrained there. (As the rod moves up and down, the whole plate flops up and down relative to the anchor point.)

In addition to sand, which settles in nodes, one can also use a substance called lycopodium powder, which is the dried spores of club moss. This substance does not settle in the nodes, but rather, it floats at the antinodes. Because lycopodium powder was sometimes used with these plates, some of the photographs show fuzzy, yellowish blobs among the nodal lines marked by the sand.

1) Patterns produced in the circular plate:

Four short radial nodes near the edge
154 Hz
 
  Two outer lobes   Three outer lobes   Four outer lobes  
  89 Hz   170 Hz   285 Hz  
 
    Two circular nodes   Three circular nodes    
  349 Hz 880 Hz  
 
One circular node with four radial nodes One circular node with six radial nodes
470 Hz 697 Hz
 
Two lobes with two circular nodes Ten lobes with five inner lobes with inner circular node Four circular nodes with eight radial nodes Five circular nodes with four radial nodes
1,120 Hz 1,618 Hz 4,462 Hz 4,654 Hz
 
One circular node Two circular nodes Three circular nodes Four circular nodes
234 Hz 734 Hz 1,691 Hz 2,719 Hz
 
Five circular nodes Six circular nodes Seven circular nodes Eight circular nodes
4,007 Hz 5,838 Hz 7,558 Hz 9,659 Hz

2) Patterns produced in the square plate:

One curved (inward) node at each corner One node at each corner (perpendicular to the diagonal), with four short nodes at a short distance from the center of each edge One continuous node around the edge, with a lobe at each corner, pinched inward at each edge, and one roughly circular node inside it. One square node at each corner, each with its sides at 45 degrees to the edges.
137 Hz 248 Hz 341 Hz 419 Hz
 
Two curved (inward) nodes at each corner, with a small circular node near the center Two curved nodes at each edge (curving inward), with one large circular node and one small circular node around the center One inward-curved node at each corner and the center of each side, with one continuous four-lobed node in the center (Each lobe points along its half of a diagonal; it resembles a cross set at 45 degrees to the sides of the square.) Essentially, two square nodes at each corner, at 45 degrees to the sides of the plate. The inner nodes flare outward as they reach the edge, and almost touch at their inner corners.
476 Hz 775 Hz 934 Hz 1,036 Hz

As part of a special project, a UCSB physics student named Kelly Janus studied the vibrational modes of the square plate. She found 35 modes and took photographs of them. You can see those here.

3) Patterns produced in the violin-shaped plate:

Pattern formed at 380 Hz Pattern formed at 586 Hz Pattern formed at 625 Hz Pattern formed at 678 Hz Pattern formed at 800 Hz Pattern formed at 1,036 Hz Pattern formed at 1,210 Hz
380 Hz 586 Hz 625 Hz 678 Hz 800 Hz 1,036 Hz 1,210 Hz
 
Pattern formed at 1,324 Hz Pattern formed at 1,428 Hz Pattern formed at 1,592 Hz Pattern formed at 1,648 Hz Pattern formed at 1,879 Hz Pattern formed at 2,070 Hz Pattern formed at 2,357 Hz
1,324 Hz 1,428 Hz 1,592 Hz 1,648 Hz 1,879 Hz 2,070 Hz 2,357 Hz
 
Pattern formed at 2,750 Hz Pattern formed at 2,947 Hz Pattern formed at 3,277 Hz Pattern formed at 3,552 Hz Pattern formed at 3,756 Hz Pattern formed at 3,905 Hz Pattern formed at 4,080 Hz
2,750 Hz 2,947 Hz 3,277 Hz 3,552 Hz 3,756 Hz 3,905 Hz 4,080 Hz