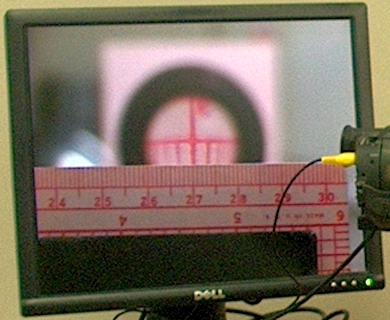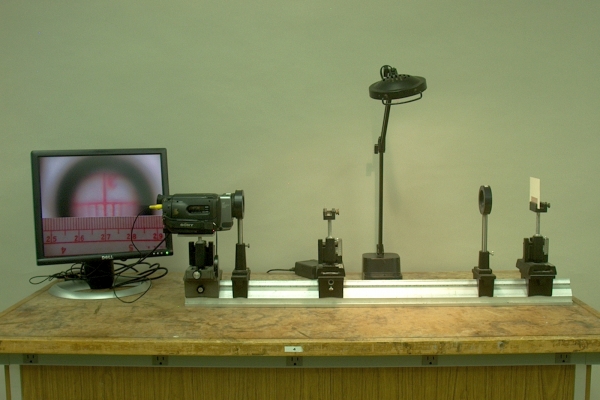
On an optics rail are five carriages holding, from left to right, a video camera, a 200-mm-focal-length eyepiece lens (ocular), a millimeter scale with a white card (reticle), a 100-mm-focal-length objective lens, and a millimeter scale with a white card, as an object. The camera is set to focus at infinity, and zoomed to provide an angle of view comparable to that of the human eye, or otherwise to provide a view that makes clear the operation of the apparatus. Thumbscrews on the carriages allow you to make fine adjustments to the positions of the reticle and the object in both the vertical and horizontal directions. A lamp provides illumination of the reticle and object, in case this is necessary. As set up in the photograph, the microscope shows an approximately 3:1 size ratio between the image formed by the object, and that of the reticle, essentially the magnification of the objective (not the overall magnification; vide infra). The video display, shown facing front for purposes of photography, normally faces the rear of the table, so that you can see the image as you manipulate the apparatus. A tee connected to the camera output allows connection to the projector in the lecture hall.
A microscope allows you to examine objects that are too small to see with the naked eye. In a compound microscope, such objects are placed close to the objective lens of the instrument, and viewed through an eyepiece, or ocular. The apparatus in the photograph above is a thin lens version of such an instrument. (In most microscopes, the lenses comprise multiple elements, to correct for various types of aberration.) The object, whose height is h, is placed just outside the focal length of the objective lens, which forms a real, inverted image, with height h′, at a distance s from the focal point, fob, on the other side of the objective (so at a distance fob + s from the lens; s is sometimes called the tube length). The lateral magnification of this image, m, equals h′/h, which equals -s/fob. In the apparatus above, s is about 32.5 cm, so m = 32.5/10.0 = 3.25. The microscope is constructed so that the point at which this image forms is at the focal point, F1, of the eyepiece, or a distance of feye behind it. The eyepiece acts as a simple magnifier, producing from the image at F1 parallel rays, which then enter the eye (in this case the camera) to produce an image at infinity. Normally, the angular magnification of the eyepiece (or a simple magnifier) should be the near point of the eye, which is usually taken as 25 cm, divided by the focal length of the magnifier, in this case 25 cm/20 cm, or 1.25. The overall magnification is the product of the lateral magnification of the objective and the angular magnification of the eyepiece, which in this case would be 3.25 × 1.25 = 4.1. We may estimate the near point of the camera, and the overall magnification, by noting the relative sizes of the image with and without the ocular:
If we measure the distance between the 25-cm and 29-cm marks on the reticle in the two photographs above (both were taken from the same vantage point and at the same camera setting, and are exactly the same size), we find that the ocular affords a magnification of about 1.6. With an eyepiece focal length of 20 cm, this indicates a camera near point of 32 cm, and a total magnification of 3.25 × 1.6 = 5.2.
The near point of the eye is the shortest distance at which one can hold an object in front of the eye and still obtain a clear image. Placed any closer than this, an object appears blurry. This puts a limit on how large we can make a small object appear by bringing it close to the eye. If we place in front of the eye a lens whose focal length, f, is shorter than the near point distance to the eye, and then place an object at the focal length of that lens, the lens produces a virtual image, at infinity, of the object. (The diverging rays from the object at the focal point, emerge from the other side of the lens as parallel rays.) For a given object height, h, and a distance from the lens, s, the angle subtended by the object is given by tan θ = h/s. For small angles tan θ ≈ θ, and we may take the angle as θ = h/s. For the magnifying glass working as described here, θ = h/25 cm, θ′ = h′/f, and M = θ′/θ = 25/f. So, for instance, if we use a magnifying glass having a focal length of 10 cm, M = 25/10 = 2.5. (You can also use a magnifying glass by placing the object slightly closer to the lens than its focal length. This forms a virtual image at the near point, and adds 1 to the expression for M, which then equals (25/f) + 1.)
Referemces:
1) Halliday, David and Resnick, Robert. Physics, Part Two, Third Edition (New York: John Wiley and Sons, 1977), pp. 980-981.
2) Jenkins, Francis A. and White, Harvey E. Fundamentals of Optics (New York: McGraw-Hill Book Company, Inc., 1950), pp. 161-165.