A video of this demonstration is available at this link.
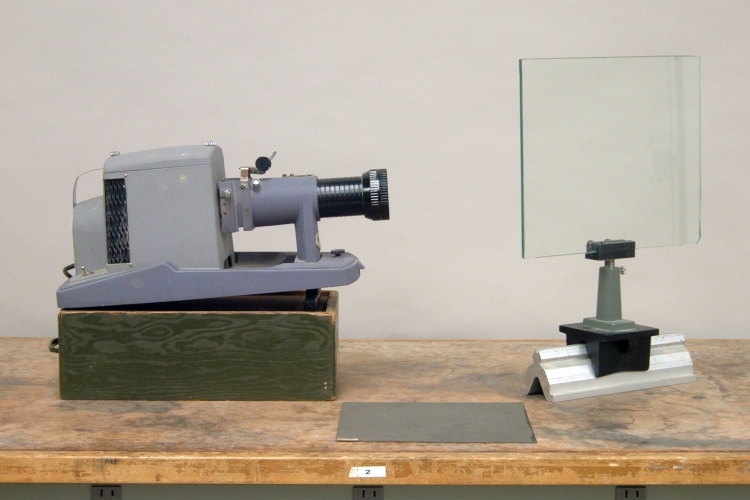
A projector is aimed to throw a spot on a screen. A glass plate sits in front of the projector, set at Brewster’s angle with respect to the path that the light takes from the projector to the screen. The glass plate reflects some of the incident light, which produces a spot on the chalkboard (or wall, depending on the exact location and orientation of the demonstration; the plate is set so that the side that faces the projector faces away from the class). If you set the polarizer so that its easy axis is vertical, and then place it in the path of the reflected light, the spot remains. If you then turn the polarizer so that its easy axis is horizontal, the spot disappears. If you place the polarizer between the projector and the glass plate, with its easy axis vertical, the spot from the reflected light remains. If you turn the polarizer so that its easy axis is horizontal, the spot from the reflected light disappears. Also, the spot on the screen becomes slightly brighter (vide infra).
When light is incident on the surface of a transparent medium at a special angle called the polarizing angle, denoted θp, the light reflected from the surface is polarized in the direction perpendicular to the plane on which the incident and reflected rays lie. This angle is also known as Brewster’s angle, after Sir David Brewster, who discovered the law that bears his name, and which gives the tangent of this angle as the ratio of the indices of refraction of the two media (the medium on whose surface the light is incident, and the medium through which the light travels to reach that surface). That is, tan θp = n2/n1, where n2 is the index of refraction of the medium on whose surface the light is incident, and n1 is the index of refraction of the medium through which the light travels to reach the second medium. This ratio is often expressed as n. If the first medium is air, n1 is so close to 1 (1.0003, at 1 atm and 20°C) that, essentially, n = n2.
This law arises from the fact that when light is incident on a medium at this special angle, the reflected ray and the refracted ray are perpendicular to each other. Since the angles of incidence and reflection equal, if we call the angle of refraction θr, we have θp + θr = 90°. Snell’s law gives n1 sin θp = n2 sin θr. Substituting for θr gives n1 sin θp = n2 sin (90° - θp) = n2 cos θp, or tan θp = n2/n1, or, as noted above, tan θp = n.
Since the glass plate in this demonstration is vertical, the plane of incidence is horizontal, and when the light from the projector is incident on the plate at Brewster’s angle, the light reflected from the plate is vertically polarized. If you place the polarizer in the path of the reflected light, with the easy axis vertical, the spot from the reflected light remains. If you then turn the polarizer so that its easy axis is horizontal, and thus block the vertically polarized light, the spot disappears.
The plate reflects some of the vertically polarized light coming from the projector, and transmits the rest to the screen. It allows essentially all of the horizontally polarized light through. When you place the polarizer between the projector and the glass plate, with the easy axis vertical, you see both the spot from the reflected light and the spot on the screen from the transmitted light. When you turn the polarizer so that its easy axis is horizontal, the spot from the reflected light disappears, and you see on the screen a spot from the horizontally polarized light. Enough of the vertically polarized light is reflected by the plate, that the amount of vertically polarized light that reaches the screen is noticeably less than the amount of horizontally polarized light that reaches the screen. As a result, when you turn the polarizer so that its easy axis is horizontal, the spot on the screen becomes slightly brighter than it was when the polarizer’s easy axis was in the vertical position.
We could place a series of plates in the path of the projector, all at Brewster’s angle (so all parallel). Since each plate would reflect some of the incident vertically polarized light, and transmit virtually all of the incident horizontally polarized light, with enough plates we could thus remove most, or almost all, of the vertically polarized light from the transmitted beam, leaving mostly, or only, horizontally polarized light to reach the screen.
References:
1) Halliday, David and Resnick, Robert. Physics, Part Two, Third Edition (New York: John Wiley and Sons, 1978), pp.1073-5.