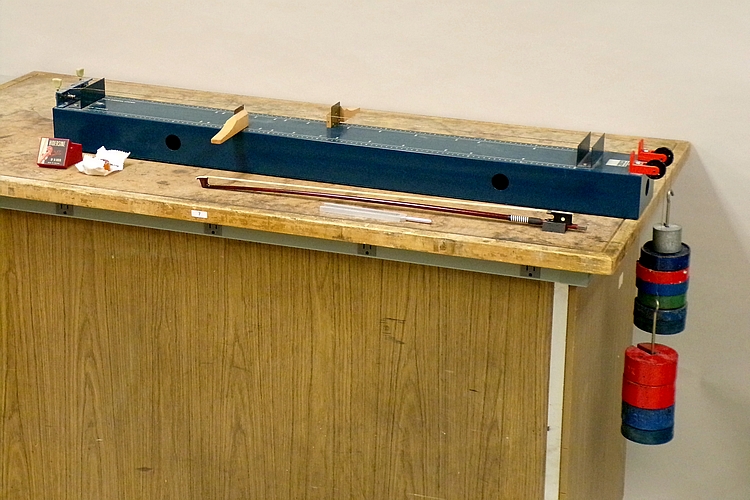
Two steel wires of different diameters run the length of a hollow rectangular box. One end of each wire is fixed to a tuning peg at one end of the box, where it runs over the edge of a metal plate to the other end of the box. There, each wire runs over the edge of a second plate and then over a pulley, to a mass hanger attached at the end. The hanging masses apply tension to the wires, which you can adjust by varying the mass. By means of two bridges, shown above one-third of the way from the left end of one wire and at the middle of the other, you can shorten the vibrating length of wire, or divide the wire into two vibrating sections whose lengths have specific ratios. You can either pluck the wires or use the violin bow to set them vibrating. The tuning fork provides a pitch reference (C = 256 Hz).
The apparatus in the photograph above is a dual monochord. A monochord is an instrument that has a single string whose vibrating length and tension you can vary. If you pluck or bow the string, it sounds a certain pitch, which depends on the vibrating length, tension and linear density (mass per unit length) of the string. Monochords date to ancient times. Perhaps the most famous experiments performed with a monochord are those by Pythagoras. His monochord had a moveable bridge, which divided the string into two sections. He found that if he placed the bridge so that the lengths of the two sections were in whole number ratios, the two pitches they produced sounded pleasing; they formed some musical interval. Probably because it allows one to study the physics of a vibrating string by observing the sound it produces, it is common for people to refer to such an instrument as a sonometer. As noted above, the apparatus in this demonstration has two wires, whose overall vibrating length is determined by the distance between the metal plates at their ends, which is 1.00 meters. It resembles the type of instrument Pythagoras used, in that it has for each string a moveable bridge, which you can use to divide the string into sections whose lengths have specific whole number ratios. You can thus form different musical intervals and show how these ratios correpond to them. You can also use the bridge to shorten the string by a certain amount, to see how much this increases the pitch. If you wish, you can also touch the string lightly at a particular point, to produce the harmonic that has a node there. For example, lightly touching the string in the middle produces the second harmonic (an octave above the fundamental), touching it at one-third the length from the end produces the third harmonic (an octave and a fifth above the fundamental), one-quarter length from the end produces the fourth harmonic (two octaves above the fundamental), etc. You can sound these harmonics by plucking the string, but it is probably more effective to use the violin bow.
The violin bow should be tightened and prepared with rosin (shown near the left end of the sonometer) before class. If you need to adjust the tension, you can do so by turning the screw at the end of the bow. (Do not overtighten the screw; the bow should still have some curvature.) If you feel that the bow is slipping too much, you can rub the cake of rosin along the hair.
As shown on the page for demonstration 40.54 – Waves on cord, tubing, spring, the velocity of a wave on a linear elastic medium such as a wire is
v = √(T/μ)
where T is the tension in the wire, and μ is the linear density of the wire. For a wave traveling in a medium, its velocity, frequency and wavelength are related by
v = νλ, so ν = (1/λ)√(T/μ)
Since a standing wave occurs any time an integral number of half wavelengths fits within the vibrating length of wire, L, we have
λ = 2L/n, and ν = (n/2L)√(T/μ)
At any frequency that satisfies this equation for a given value of n, we obtain a standing wave that has one node at each end of the vibrating length of wire, and n antinodes and n - 1 nodes between the ends. For the first harmonic, or fundamental, n = 1. If we call the frequency of the fundamental ν0, then we expect harmonics at frequencies of ν0, 2ν0, 3ν0, 4ν0, 5ν0, etc. Note that if we excite only the fundamental, then if without changing the tension, we change the length of the string so that it equals the original length divided by a whole number, the new fundamental is the original fundamental multiplied by that number. That is, if we halve the string length, we double the frequency of the fundamental, if we take one third the original length, we triple the frequency, if we take one quarter the length, we quadruple the frequency, etc. If we make the new length some whole number fraction of the original length, the ratio between the new frequency and the original frequency will be the inverse of that fraction. For example, if we make the length 2/3 the original length, the new frequency is 3/2 the original frequency. If we make the string 4/5 its original length, the frequency goes up by a factor of 5/4. (Vide infra.)
The wire along the side of the box facing the front of the table has a diameter of 0.74 mm and a linear density of 3.4 g/m. With 7 kilograms hanging from the end, as shown, the tension is 68.7 N, and the frequency given by the equation above is 71 Hz. The wire along the other side of the box has a diameter of 0.34 mm and a linear density of 0.85 g/m. With 6.5 kilograms hanging from the end, as shown, the tension is 63.8 N, and the frequency given by the equation above is 137 Hz. The frequencies at which the two strings actually sound are 67 Hz and 134 Hz, respectively. These are both somewhat lower than the calculated frequencies, the first by a half step, and the second by about 0.4 half steps. They are, however, exactly an octave apart, which is convenient for operating this apparatus. You may, of course, change the tension on either string to show its effect on the frequency, and though it is convenient, the frequencies of the two strings do not necessarily need to be harmonically related.
Along each edge of the sound box is a scale marked in centimeters and millimeters. At various places along each scale are markings with note names (C, D, E, F, G, A, B) and the frequencies of the corresponding pitches. The end of the string near the pulleys is the 100-cm mark, and has on each scale the label, “C256.” The two octaves below this are C128 and C64. The 67-Hz and 134-Hz pitches mentioned above are not quite a half step sharp relative to these. So with this arrangement, according to the physics pitch standard, the instrument is pitched roughly in C-sharp. The scale on the edge near the front of the table bears the label, “DIATONIC SCALE,” and the scale on the other edge bears the label,“EQUALLY TEMPERED SCALE.” A brief explanation of this follows, but first a note on the overtone series is in order.
The overtone series
The experiments of Pythagoras and others with monochords (and, most likely, also pipes), revealed to them what we now call the overtone series, which has tremendous significance to music. It provides the basis for musical scales and intervals. The overtone series is, of course, the harmonic series, but with a slight difference in nomenclature. In both series, the lowest-frequency member is the fundamental. It is common to refer to the second harmonic as the first overtone, the third harmonic as the second overtone, etc. As noted on the page for demonstration 44.12 – Ear model, we hear differences between pitches according to the ratios of their frequencies. That is, we hear any pair of pitches whose frequencies are in a particular ratio as being the same musical interval apart. As noted above, the harmonics are successive integer multiples of the frequency of the fundamental. The interval between any two members of a harmonic series – two pitches whose frequencies are in whole number ratios – will sound pleasant. If the frequencies of two pitches cannot be expressed as a whole-number ratio, then the pitches are not harmonically related, and their interval sounds out of tune. As we go up in the harmonic series, the frequency ratios of neighboring harmonics become successively smaller, and thus, so do the musical intervals between them. A frequency ratio of 2:1, which we have between the second harmonic and the fundamental, corresponds to a musical interval of an octave. For the first three octaves in the harmonic series, the frequencies and intervals are as in the following table.
n ν Interval from Previous Harmonic Interval from Fundamental 1 ν0 Unison (perfect prime)
Unison (perfect prime)
2 2ν0 Octave Octave 3 3ν0 Perfect fifth Octave + One perfect fifth 4 4ν0 Perfect fourth Two octaves 5 5ν0 Major third Two octaves + One major third 6 6ν0 Minor third Two octaves + One perfect fifth 7 7ν0 Minor third* Two octaves + One minor seventh* 8 8ν0 Major second* Three octaves Intervals are named according to the number of scale degrees that separate the two pitches that make them. Going up from the first step of a major scale, the tonic, the rest of the scale steps are, in succession, a major second, major third, perfect fourth, perfect fifth, major sixth, major seventh and an octave above the tonic. The intervals between neighboring scale degrees are major and minor seconds – whole steps and half steps, respectively.Within the first three octaves of the harmonic series, we have the major third, which corresponds to a frequecy ratio of 5:4, the perfect fourth, whose ratio is 4:3, the perfect fifth, whose ratio is 3:2, and the octave, at 2:1. The asterisks on the intervals for the seventh and eighth harmonics are there because though they are close, they do not equal the ratios by which we normally define these intervals (vide infra).
The building of scales and intervals from the overtone series is a rich and fascinating subject. One can arrive at a C major scale by going through a cycle of fifths and octaves. For example, if we start at F and go up in perfect fifths, we get C, G, D, A, E and B. (We can insert downward octaves in spots to keep everything within two octaves.) If we wish to fill in all the half steps, we can arrive at the chromatic scale by going around the circle of fifths: F-C-G-D-A-E-B-F#-C#-G#-D#-A#-E#(F) (or we could go from C to B#(C)). We could go up 12 fifths, which in principle should be equivalent to going up seven octaves, or we could alternate going up in fifths with going down in octaves, which amounts to the same thing. We should end up either on a note seven octaves above our starting note, or on the same note at which we started. For this to happen, though, (3/2)12 must equal 27, but (3/2)12 = 129.75, and 27 = 128.00. This frequency ratio equals 1.0136, which corresponds to a pitch difference of about 23.5 cents, almost a quarter of a half step (vide infra). This discrepancy is called the comma of Pythagoras. If we explore other interval combinations that we might think should be equivalent, we find other discrepancies as well. If we try to tune all of the intervals within a major scale so that they sound in tune, then, we find that this is not such an easy task. A system according to which we tune the steps of a scale is called a temperament.
Pythagoras used a system based on fifths and octaves, in similar fashion to what is described above, to obtain all the steps in the chromatic scale. One fifth – called the wolf fifth – ends up being out of tune by the amount that corresponds to the comma of Pythagoras. In addition, the major thirds are badly out of tune. For example, the major third up from the tonic (C to E) has a frequency ratio of 81:64 (1.2656) instead of 5:4 (1.2500). This difference, about 21.5 cents, is called the comma of Didymus.
In the system called just intonation, all intervals are derived from the natural fifth, natural major third and natural octave, i.e., those shown in the table above. For example, a minor third is a perfect fifth minus a major third, or 3:2 × 4:5, or 6:5, a major second is a perfect fifth minus a perfect fourth, or 3:2 × 3:4, or 9:8, a major sixth is an octave minus a minor third, or 2:1 × 5:6, or 5:3, etc. This system is considered to provide the most pleasant-sounding major triads (built on the first, fourth and fifth steps of the scale), because it puts them all in 4:5:6 ratios. (Note that the fourth, fifth and sixth harmonics together make up a major triad. This property is probably why the German name for just intonation is reine or natürliche Stimmung, for “pure” or “natural voicing.”) It is this system to which the label “DIATONIC SCALE” on the sound box refers. Plucking or bowing the open string (i.e., no bridge under it) gets the fundamental, which we can call C. If you wish to sound a different note, you shorten the string in inverse proportion to the frequency ratio between that note and C. For example, assuming that the open string sounds C256, if you wish to sound A426.7, the frequency ratio is 426.7/256 = 1.667. The reciprocal of this is 0.6000, so if you place the bridge at 60.00 cm and then pluck or bow the string between the bridge and the tuning peg, it will sound A426.7. If you wish to sound F341.5, the ratio is 341.5/256 = 1.3340, and the reciprocal is 0.7496. If you set the bridge at 74.96 cm and then pluck or bow between the bridge and the tuning peg, it will sound F341.5. For some reason, the mark for D288 is at the wrong location. A label shows the correct location. Except for this error, the string lengths for the various notes are calculated according to the two examples above. The frequencies of the steps of the diatonic scale are in the following ratios relative to the tonic:
The C Major Scale According to Just Intonation Ratio to C = 256 Frequency/Hz String Length, cm C 1 256 100.00 D 9/8 288 88.89 E 5/4 320 80.00 F 4/3 341.3 75.00 G 3/2 384 66.67 A 5/3 426.7 60.00 B 15/8 480 53.33 C 2 512 50.00 For higher octaves, multiply the frequency ratio by the appropriate power of two. You can use these ratios to calculate the frequencies that correspond to the 67-Hz and 134-Hz fundamentals that you get with the masses shown. If you use different masses, then you can use them to calculate frequencies that correspond to the fundamentals that you get with those masses.
Unfortunately, just temperament has serious disadvantages that prevent its use for tuning instruments, but it does provide a useful reference for calculating frequency ratios for intervals that we hear as being “pure” or “natural.” Intervals having these frequency ratios are called just-tuned or just. The ratios given above for the major third (5:4), perfect fourth (4:3), perfect fifth (3:2) and octave (2:1) correspond to those for just-tuned intervals. A just minor second has a frequency ratio of 16:15, major second 9:8, minor third 6:5, minor sixth 8:5, major sixth 5:3, minor seventh 16:9, and major seventh 15:8. Note that in the just-tuned scale, three of the whole steps have a ratio of 9:8, and the other four have a ratio of 10:9; they are not all the same size.
The biggest disadvantage of just intonation is that, while music played in the key in which the instrument is tuned sounds quite pleasant, music played in other keys does not sound as well in tune. If you change the tonic, the frequency ratios of all the different pitches relative to the new tonic are different from the corresponding ratios in the original key. These differences, which cause the music to sound out of tune, are not so bad if the new key is closely related, harmonically, to the original key. For example, if an instrument is tuned in C major, music in C major sounds quite good. If the music modulates to G major (one sharp) or F major (one flat), it will not sound as good as it did in C major, but it will still not sound unpleasant. The more harmonically distant the new key is from C major, however, the worse the intonation, so that music played in any key that is not closely related to C major sounds rather bad. As one gets further from the original key, the tuning errors tend not to spread uniformly, but rather to concentrate in a few intervals. A temperament that has these characteristics is called an open temperament.
If one wishes to tune an instrument so that it can play reasonably well in all keys, one must make certain compromises to spread the tuning errors so that no one interval or set of intervals becomes too badly out of tune. A temperament that accomplishes this is called a closed temperament. A closed unequal temperament is one in which not all the half steps in the chromatic scale are the same size. Perhaps the most popular of this type of temperament are those by Andreas Werckmeister (1645-1706). In these temperaments, the errors vary from key to key, so that in some keys the music sounds quite good, while in others it is not as good, but in no key is it unpleasant. Another type of closed temperament is equal temperament, in which the octave is divided into 12 equal half steps. While certain intervals, especially thirds and sixths, are noticeably out of tune, the intonation of all the other intervals is fairly good, and the overall intonation is good enough to allow one to play music. The main advantage of equal temperament is that the tuning errors are identical in all keys, so that no matter how far music modulates from its original key, it will still sound good. It is this system to which the label “EQUAL TEMPERAMENT” on the sound box refers.
Dividing the octave into 12 equal half steps means that each half step has a frequency ratio of 12√2 = 1.0595. As the text above shows, to add intervals we must multiply frequency ratios. If we add twelve half steps, we get (12√2)12 = 2, which is one octave, as it should be. If we express frequency ratios as logarithms, then we can add them directly. The interval of a half step is defined as 100 cents, and 12 of them add to 1,200 cents; one cent equals one one-hundredth of a half step. Thus, to find the difference, I, between two pitches in cents, if their frequencies are f1 and f2, we have:
I = 1,200 [log( f1/f2)/log 2]
If we don’t know the frequencies of two pitches, but know their ratios relative to a third pitch, then we can put those ratios in for f1 and f2 in the equation above. For example, the difference between an equal-tempered half step and a just-tuned half step is 1,200 [log(1.0595/[16/15])/log 2] = 1,200 [log(1.0595/1.0667)/ log 2] = 1,200 (-2.9429 × 10-3/0.3010) = -11.73 cents. The equal-tempered half step is 11.73 cents narrower than the just-tuned half step. This is how the commas noted above were calculated. If we have an interval, I, expressed in cents, we can find the frequency ratio by:
f1/f2 = 2(I/1200)
As described above for the diatonic (just-tuned) scale, by placing the bridge at the appropriate locations along one of the strings, you can sound pitches that correspond to those of a scale tuned according to equal temperament. The table below gives the frequency ratios of the scale steps relative to the tonic, and their string lengths, for an equal-tempered scale. As with the diatonic scale, the mark for D287.35 is in the wrong place. A label shows the correct location.
The C Major Scale According to Equal Temperament Ratio to C = 256 Frequency/Hz String Length, cm C 1 256 100.00 D 1.1225 287.4 89.09 E 1.2599 322.5 79.37 F 1.3348 341.7 74.92 G 1.4983 383.6 66.74 A 1.6818 430.5 59.46 B 1.8877 483.3 52.97 C 2 512 50.00 As above, for the higher octaves, multiply the frequencies by the appropriate power of two. Again, you can use these ratios to calculate the frequencies that correspond to the 67-Hz and 134-Hz fundamentals that you get with the masses shown. If you use different masses, then you can use them to calculate frequencies that correspond to the fundamentals that you get with those masses.
References:
1) Resnick, Robert and Halliday, David. Physics, Part One, Third Edition (New York: John Wiley and Sons, 1977), pp. 412-413.
2) Berg, Richard E. and Stork, David G. The Physics of Sound (Englewood Cliffs, New Jersey: Prentice-Hall, Inc., 1982), pp. 67-75, 319-320, 349-351.
3) Apel, Willi. Harvard Dictionary of Music (Cambridge, Massachusetts: Harvard University Press, 1955) pp. 359-363 (Intervals, and Intervals, Calculation of), pp. 384-385 (Just intonation).