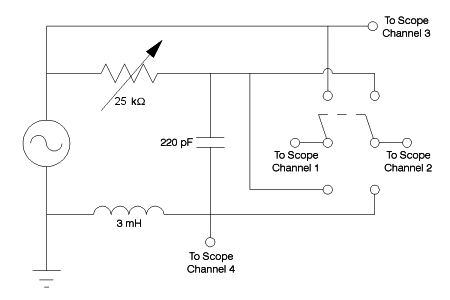
A potentiometer, a capacitor, an inductor and a signal generator are connected in series. On the oscilloscope, you can read the voltages across the signal generator, the potentiometer, the capacitor and the inductor. (Two versions of the circuit are available. Shown above is the circuit that allows you to display simultaneously the voltages across the signal generator, the inductor and either the capacitor or the potentiometer, which you choose via the switch at right in the photograph. In the other circuit, the switch selects either the capacitor or the inductor.) The edges of a square wave produced by the signal generator excite oscillations in the circuit, which decay over time. By changing the resistance, you can change the damping in the circuit, and thus change the decay time.

Demonstration 72.63 -- LRC circuit: phase differences, resonance, shows the behavior of an LRC circuit driven by a sinusoidal voltage. In particular, it allows you to show what happens when you drive the circuit at its resonant frequency, and to compare this behavior to what happens when you drive it at frequencies above and below this frequency. In this demonstration, the signal generator is set to produce a square wave whose frequency is much lower than the resonant frequency of the circuit. Each edge of the square wave sets the circuit oscillating, and each half cycle is long enough to allow many oscillations at the natural frequency of the system. Applying a square wave across the LRC circuit is analagous to alternately connecting the ends of the circuit across a DC power supply and shorting them.

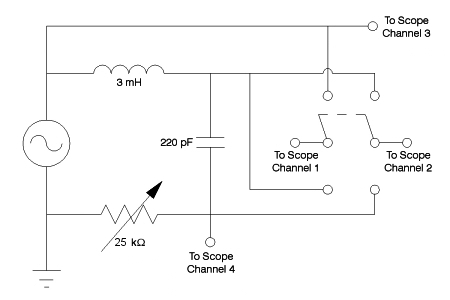
The schematic above shows the circuit (circuit B). A .pdf version of this schematic is available here to download and show on the data projector. Channel three on the oscilloscope shows the voltage applied by the signal generator to the circuit, and channel four shows the voltage across the inductor. Channels one and two are connected via a double-pole, double-throw switch to the ends of either the capacitor (switch down) or the resistor (switch up). The oscilloscope subtracts the signal on channel two from that on channel one, and displays the difference on a separate trace. This allows you to show the voltages across the capacitor and the inductor simultaneously, and to switch between the capacitor and the resistor.

The original circuit, which is still available, had the resistor and the inductor in the opposite positions, with oscilloscope channel four showing the voltage across the resistor, and the switch connected to the capacito and the inductor, so that you can display the voltage across the resistor simultaneously with that across the inductor or the capacitor (circuit A):

An overhead transparency of this circuit is also available, or you can dowload the .pdf file here. Traces obtained with both circuits appear below.

Please note: because of the phase relationships of the voltages across the three components, circuit B offers some advantage over circuit A. For this reason circuit B is now the default. Traces for both are included here for illustration. If you would prefer circuit A, please state this when you request this demonstration.

To analyze the behavior of this circuit, we can find an expression for the current flowing in it. To do this, we recognize that for the shorted loop, the sum of the potentials across all the components must equal zero, or

iR + L(di/dt) + q/C = 0

Actually, for the oscilloscope traces shown below, the applied square wave wave was symmetrical about zero. So there is a positive DC potential applied across the ends of the loop during the positive half cycle, and a negative DC potential applied during the negative half cycle. We will see the effect of this in the oscilloscope traces. In all cases, the voltages across the individual components must add up to the applied potential. For our analysis, however, we may use the equation above. If we note that i = dq/dt, and then substitute, we get

R(dq/dt) + L(d²q/dt²) + q/C = 0

which we can rearrange to

L(d²q/dt²) + R(dq/dt) + (1/C)q = 0

Alternatively, we can use the principle of conservation of energy. If there is no resistance, the total energy in an oscillating LC circuit is the sum of the energy stored in the inductor and that stored in the capacitor, or

U = (1/2)Li² + (1/2)(q²/C)

With no resistance in the circuit, this energy is constant, and

dU/dt = Li(di/dt) + (q/C)(dq/dt) = 0

since i = dq/dt, di/dt = d²q/dt², and

L(d²q/dt²) + (1/C)q = 0

With a resistor in the circuit, however, energy is dissipated as heat in the resistor, so

dU/dt = -i²R

and

Li(di/dt) + (q/C)(dq/dt) = -i²R

rearranging, and noting again that i = dq/dt, we have, as above,

L(d²q/dt²) + R(dq/dt) + (1/C)q = 0

This equation is exactly the same form as that for the damped mass-spring oscillator. (See demonstration 40.33 -- Mass-springs damped in oil, water.) In these two equations, q corresponds to x, i (= dq/dt) corresponds to v (= dx/dt), L corresponds to m, and C corresponds to 1/k. The magnetic energy in the inductor ((1/2)Li²) is analogous to the kinetic energy of the mass ((1/2)mv²), and the electrical energy in the capacitor ((1/2)(q²/C)) is analogous to the potential energy in the spring ((1/2)kx²). This suggests that in some sense, the behavior of an inductor is similar to that of a mass in a mass-spring system, and the behavior of a capacitor is similar to that of a spring in a mass-spring system. Just as the energy in a mass-spring system is constantly being converted from potential energy in the spring to kinetic energy in the mass, and back, so is the electrical energy stored in the capacitor constantly converted to magnetic energy in the inductor, and back.

The characteristic equation that corresponds to the last equation above is Lr² + Rr + 1/C = 0. The roots to this equation are [-R ±√(R² - 4L/C)]/2L. If we divide through by L, and substitute 2b for (R/L) and ω² for 1/LC, the equation becomes

(d²q/dt²) + 2b(dq/dt) + ω²q = 0

and the roots become r₁ = -b + √(b² - ω²) and r₂ = -b + √(b² - ω²). The radical equals √(R²/4L² - 1/LC). This gives rise to three different situations, depending on the relative sizes of b and ω, or R² and L/C:

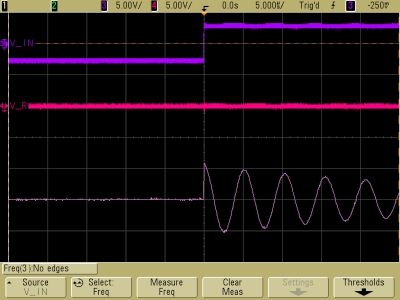
1) If b² - ω² < 0, the system is said to be underdamped. In this case, the roots are complex and unequal. If we let ω² - b² = α, the roots are r₁ = -b + αi and r₁ = -b - αi. This leads to the solution

q = e^-bt(C₁ cos αt + C₂ sin αt).

If we set C₁ = A cos φ and C₂ = A sin φ (where A = √(C₁² + C₂² and tan φ = C₂/C₁), we can use the trigonometric identity

A cos (αt - φ) = A cos φ cos αt + A sin φ sin αt

to obtain

q = Ae^-bt cos (αt - φ)

This equation describes damped oscillation. This is similar to simple harmonic motion whose frequency, in radians per second, is α, and whose period is T = 2π/α, except that the amplitude is not constant. Because of the factor e^-bt (= e^-(R/2L)t), which is called the damping factor, the amplitude (which equals Ae^-(R/2L)t), decays over time. As noted above, b, in the damping factor, equals R/2L. The greater the resistance (or the smaller the inductance), the greater the damping, and the more quickly the oscillations decay. The resistance plays a similar role to that of friction in the damped mass-spring oscillator. We can see from the equation above, that q oscillates between the two curves q = Ae^-bt and q = -Ae^-bt.

The period of oscillation, T = 2π/α, = 2π/√(ω² - b²) (= 2π/√[(1/LC) - (R²/4L²)]), is longer than that for the circuit without a resistor (an LC circuit), for which T = 2π/ω (= 2π√LC). We see that as R/2L becomes smaller, T approaches that for the LC circuit, the damping factor approaches unity, and the equation above reduces to q = A cos (ωt - φ), the equation for an ideal LC circuit with no resistance.

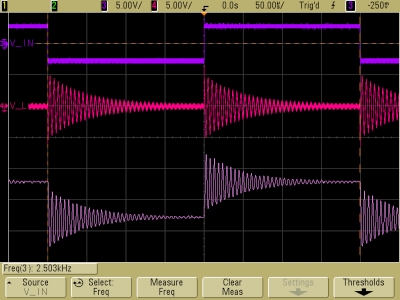
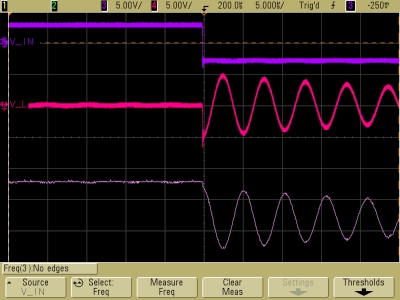
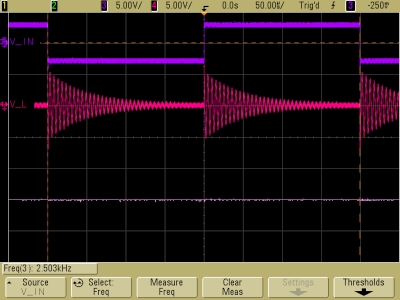
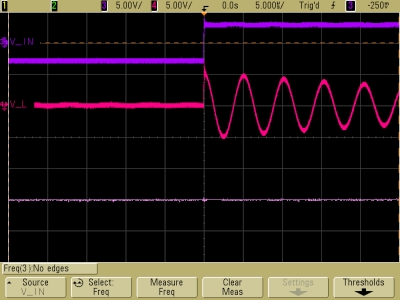
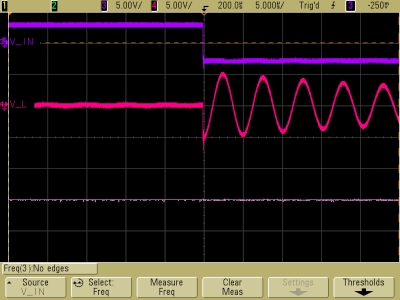
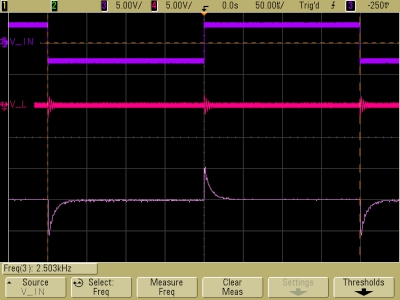
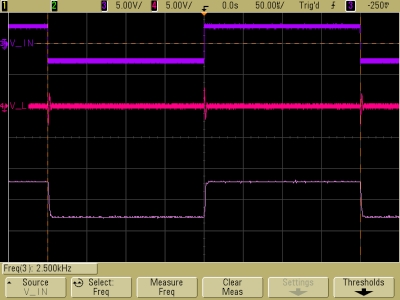
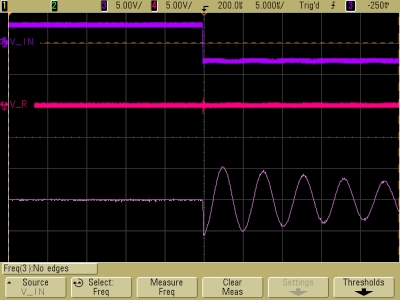
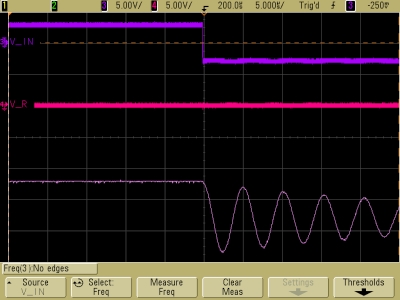
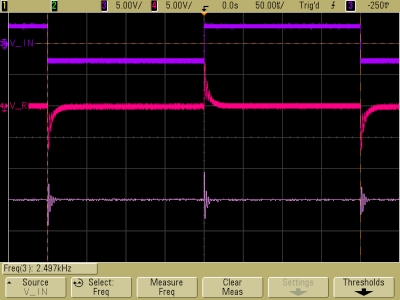
Below are oscilloscope traces for the LRC circuit with R set to zero. The applied square wave (E) has a frequency of 2.5 kHz.

E (top), VL (middle) and VC (bottom). The horizontal scale is 50 μs/division.		E (top), VL (middle) and VC (bottom), positive half cycle. The horizontal scale is 5 μs/division.		E (top), VL (middle) and VC (bottom), negative half cycle. The horizontal scale is 5 μs/division.
Note that VL always leads VC by 180 degrees.

In the panels above, the top trace is the applied square wave, the middle trace is the voltage across the inductor, and the bottom trace is the voltage across the capacitor. We see that both the voltage across the inductor and the voltage across the capacitor oscillate between two exponential curves as described above. For both the middle trace and the bottom trace, exactly 19 oscillations occur in 100 μs, which corresponds to a frequency of 190 kHz. This is close to the natural frequency of the undamped circuit, which is 1/(2π√(LC), or 1/(2π)√(3 × 10^-3 H)(2.20 × 10^-10 F) = 196 kHz. We see that the voltage across the inductor oscillates about zero, and the voltage across the capacitor oscillates about the DC level of each half cycle of the square wave. (At 2.5 kHz, the capacitive reactance in this circuit (X_C = 1/ωC) equals 290 kΩ, and the inductive reactance (X_L = ωL) equals 47 Ω. At 190 kHz, the capacitive reactance equals 3.8 kΩ, and the inductive reactance equals 3.6 kΩ; they are essentially equal. Hence the oscilloscope traces we observe.) For the panels at right, the horizontal scale has been expanded to show the first few oscillations in detail. The oscillations in the inductor lead those in the capacitor by 180 degrees. As the material on the page for demonstration 72.63 -- LRC circuit: phase differences, resonance, explains, this arises from the fact that in capacitors and inductors, the voltage and current are out of phase with each other by 90 degrees, in opposite directions. The voltage across a capacitor is V_C = q/C, and the current through it is i = dq/dt. For an inductor, the voltage is V_L = L(di/dt). For a capacitor, then, the voltage is the integral of the current, while for an inductor it is the derivative of the current. For a sinusoidal current, this means that in a capacitor the current leads the voltage by 90 degrees, and in an inductor the voltage leads the current by 90 degrees. Since the voltage across the inductor leads the current by 90 degrees, and in the capacitor the voltage lags the current by 90 degrees, the voltage across the inductor leads the voltage across the capacitor by 180 degrees. Also, as we might expect, we see that on each half cycle the phase of the start of the oscillations changes by 180 degrees.

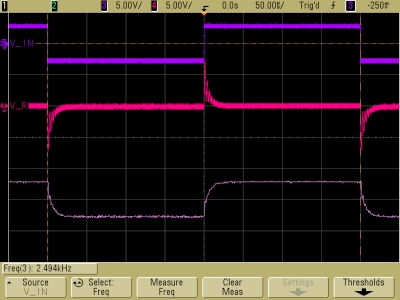
The traces below show the voltages across the inductor and the resistor:

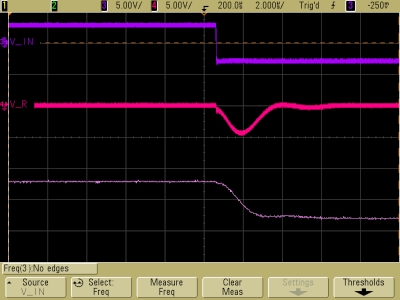
E (top), VL (middle) and VR (bottom). The horizontal scale is 50 μs/division.		E (top), VL (middle) and VR (bottom), positive half cycle. The horizontal scale is 5 μs/division.		E (top), VL (middle) and VR (bottom), negative half cycle. The horizontal scale is 5 μs/division.

2) If b² - ω² > 0, the system is said to be overdamped (or overcritically damped). The roots are real and unequal, and the solution of the differential equation is:

q = C₁e^r1t + C₂e^r2t.

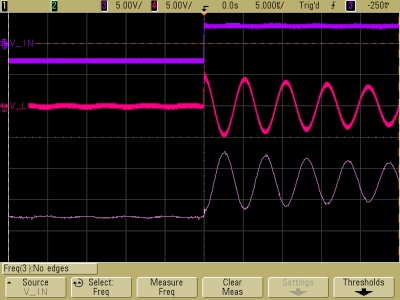
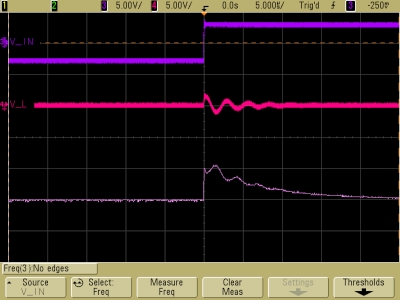
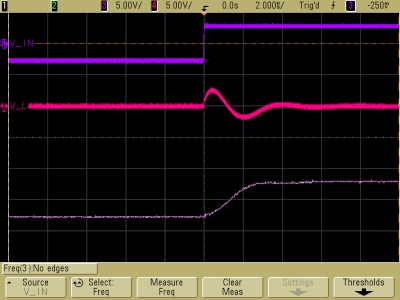
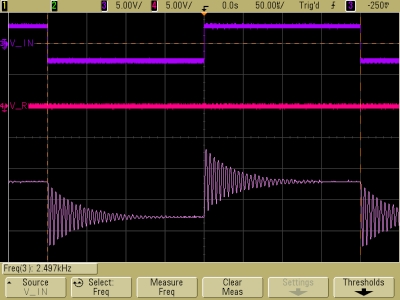
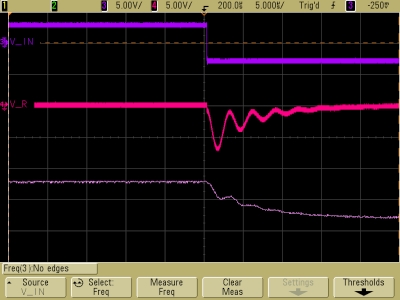
Since both r₁ and r₂ are negative, q slowly approaches zero as time increases. Depending on the conditions, the voltage may cross zero once, but no more than once, and the system does not oscillate. Below are oscilloscope traces for the circuit with R set at maximum (~25 kΩ):

E (top), VL (middle) and VC (bottom). The horizontal scale is 50 μs/division.		E (top), VL (middle) and VC (bottom), positive half cycle. The horizontal scale is 5 μs/division.		E (top), VL (middle) and VC (bottom), negative half cycle. The horizontal scale is 5 μs/division.

The bottom trace, which shows the voltage across the capacitor, shows a relatively slow rise to the DC level of the positive half cycle, and a similarly slow decay to the DC level of the negative half cycle, with no oscillation. The behavior of the inductor (middle trace) is perhaps less clear, but we see that oscillation is greatly suppressed. The traces below show the voltages across the inductor and the resistor:

E (top), VL (middle) and VR (bottom). The horizontal scale is 50 μs/division.		E (top), VL (middle) and VR (bottom), positive half cycle. The horizontal scale is 5 μs/division.		E (top), VL (middle) and VR (bottom), negative half cycle. The horizontal scale is 5 μs/division.

3) If b² - ω² = 0, the system is said to be critically damped. The roots are equal, and the solution of the differential equation is:

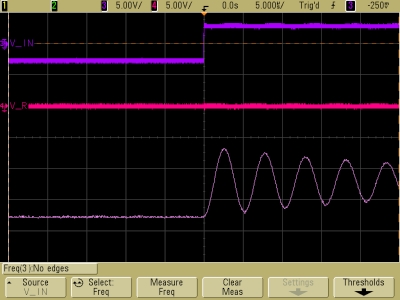
q = (C₁t + C₂)e^-ωt (or q = (C₁t + C₂)e^-bt).

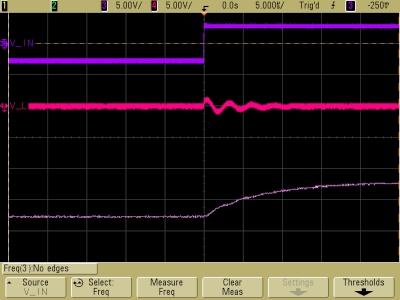
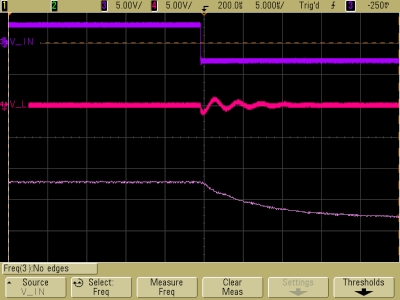
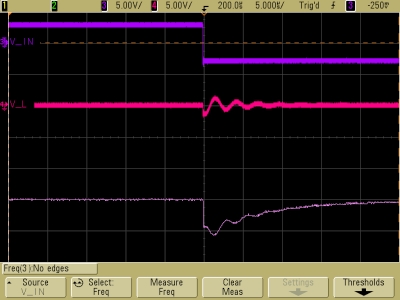
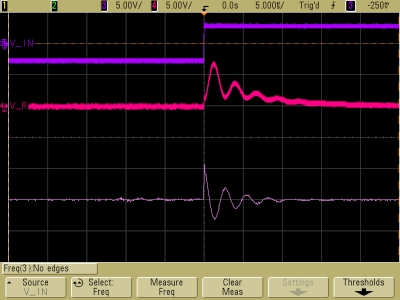
As time increases, q approaches zero (but does not cross it). Also, the oscillation dies down more quickly than it does when the system is overdamped. From the equations above, we see that critical damping obtains when R²/4L² = 1/LC, or R = 2√(L/C). For this circuit, R = 2√(3 × 10^-3 H/2.20 × 10^-10 F) = 7,400 Ω. Setting the resistor to this value appeared to provide some overdamping. For the oscilloscope traces below, R was set to about 5.5 kΩ. Note that in the panels with the expanded horizontal scale, the time base is 2 μs. (In the panels above it is 5 μs.)

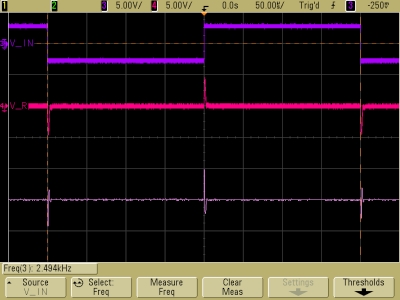
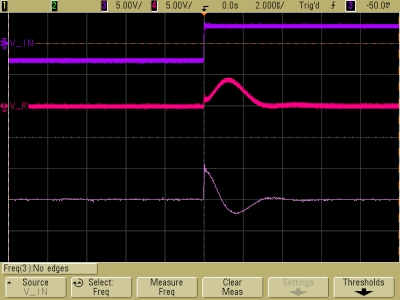
E (top), VL (middle) and VC (bottom). The horizontal scale is 50 μs/division.		E (top), VL (middle) and VC (bottom), positive half cycle. The horizontal scale is 2 μs/division.		E (top), VL (middle) and VC (bottom), negative half cycle. The horizontal scale is 2 μs/division.

The rise of the voltage across the capacitor during the positive half cycle, and its fall during the negative half cycle, are now much faster than in the overdamped circuit, and there is no oscillation. We should expect also to see one half cycle in the inductor. The portion of the trace that corresponds to the rise or fall of the voltage on the capacitor is, essentially, this half cycle, but there is a roll at the square wave edge and an overshoot at zero. The traces below show the voltages across the inductor and the resistor:

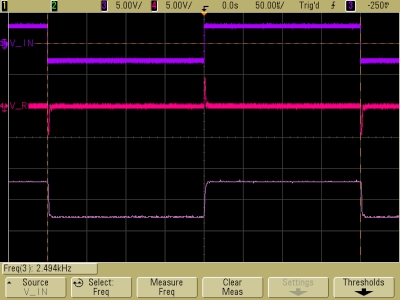
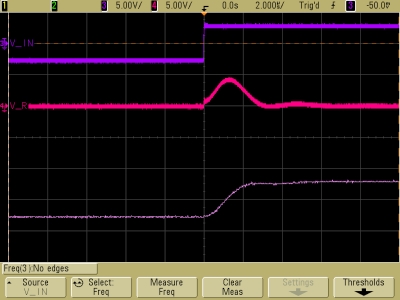
E (top), VL (middle) and VR (bottom). The horizontal scale is 50 μs/division.		E (top), VL (middle) and VR (bottom), positive half cycle. The horizontal scale is 5 μs/division.		E (top), VL (middle) and VR (bottom), negative half cycle. The horizontal scale is 5 μs/division.

As noted above, this circuit is available in two arrangements. The panels below show oscilloscope traces for the other circuit (Circuit A).

1) The underdamped circuit:

E (top), VR (middle) and VL (bottom). The horizontal scale is 50 μs/division.		E (top), VR (middle) and VL (bottom), positive half cycle. The horizontal scale is 5 μs/division.		E (top), VR (middle) and VL (bottom), negative half cycle. The horizontal scale is 5 μs/division.

E (top), VR (middle) and VC (bottom). The horizontal scale is 50 μs/division.		E (top), VR (middle) and VC (bottom), positive half cycle. The horizontal scale is 5 μs/division.		E (top), VR (middle) and VC (bottom), negative half cycle. The horizontal scale is 5 μs/division.

2) The overdamped circuit (R = 24 kΩ):

E (top), VR (middle) and VL (bottom). The horizontal scale is 50 μs/division.		E (top), VR (middle) and VL (bottom), positive half cycle. The horizontal scale is 5 μs/division.		E (top), VR (middle) and VL (bottom), negative half cycle. The horizontal scale is 5 μs/division.

E (top), VR (middle) and VC (bottom). The horizontal scale is 50 μs/division.		E (top), VR (middle) and VC (bottom), positive half cycle. The horizontal scale is 5 μs/division.		E (top), VR (middle) and VC (bottom), negative half cycle. The horizontal scale is 5 μs/division.

3) The critically damped circuit (R = 5.5 kΩ):

E (top), VR (middle) and VL (bottom). The horizontal scale is 50 μs/division.		E (top), VR (middle) and VL (bottom), positive half cycle. The horizontal scale is 2 μs/division.		E (top), VR (middle) and VL (bottom), negative half cycle. The horizontal scale is 2 μs/division.

E (top), VR (middle) and VC (bottom). The horizontal scale is 50 μs/division.		E (top), VR (middle) and VC (bottom), positive half cycle. The horizontal scale is 2 μs/division.		E (top), VR (middle) and VC (bottom), negative half cycle. The horizontal scale is 2 μs/division.

References:

1) Stewart, James. Calculus, Third Edition (Pacific Grove, California: Brooks/Cole Publishing Company, 1995), pp. 1006-9.
2) Halliday, David and Resnick, Robert. Physics, Part Two, Third Edition (New York: John Wiley and Sons, Inc., 1978), pp. 844-8.
3) Young, Hugh D., University Physics, Eighth Edition (Reading, Massachusetts: Addison-Wesley Publishing Company, 1992), pp. 881-4.