SUPERSTRINGS! M-theory 

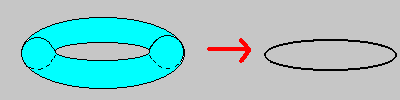
M-theory is described at low energies by an effective theory called 11-dimensional supergravity. This theory has membrane and 5-branes as solitons, but no strings. How can we get the strings that we've come to know and love from this theory? We can compactify the 11-dimensional M-theory on a small circle to get a 10-dimensional theory. If we take a membrane with the topology of a torus and wrap one of its dimensions on this circle this will become a closed string! In the limit where the circle becomes very small we recover the Type IIA superstring.

membrane

How do we know that M-theory on a circle gives the IIA superstring, and not the IIB or Heterotic superstrings? The answer to this question comes from a careful analysis of the massless fields that we get upon compactification of 11-dimensional supergravity on a circle. Another easy check is that we can find an M-theory origin for the D-brane states unique to the IIA theory. Recall that the IIA theory contains D0,D2,D4,D6,D8-branes as well as the NS fivebrane. The following table summarizes the situation:
 
M-theory on circle IIA in 10 dimensions
Wrap membrane on circle IIA superstring
Shrink membrane to zero size D0-brane
Unwrapped membrane D2-brane
Wrap fivebrane on circle D4-brane
Unwrapped fivebrane NS fivebrane
The two that have been left out are the D6 and D8-branes. The D6-brane can be interpreted as a "Kaluza Klein Monopole" which is a special kind of solution to 11-dimensional supergravity when it's compactified on a circle. The D8-brane doesn't really have clear interpretation in terms of M-theory at this point in time; that's a topic for current research!

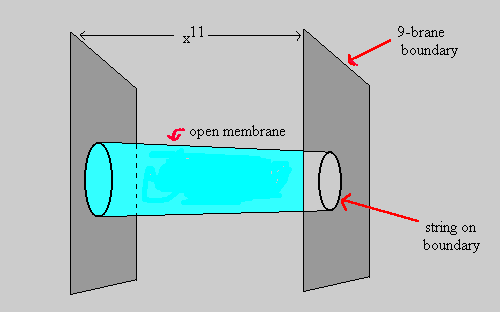
We can also get a consistent 10-dimensional theory if we compactify M-theory on a small line segment. That is, take one dimension (the 11-th dimension) to have a finite length. The endpoints of the line segment define boundaries with 9 spatial dimensions. An open membrane can end on these boundaries. Since the intersection of the membrane and a boundary is a string, we see that the 9+1 dimensional worldvolume of the each boundary can contain strings which come from the ends of membranes. As it turns out, in order for anomalies to cancel in the supergravity theory, we also need each boundary to carry an E8 gauge group. Therefore as we take the space between the boundaries to be very small we're left with a 10-dimensional theory with strings and an E8 x E8 gauge group. This is the E8 x E8 heterotic string!

M-theory with boundaries

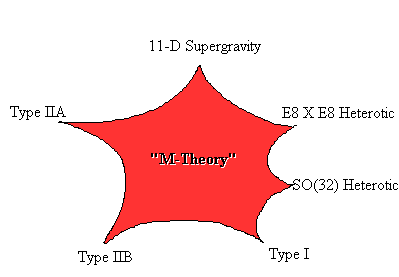
So given this new phase 11-dimensional phase of string theory, and the various dualities between string theories, we're led to the very exciting prospect that there is only a single fundamental underlying theory -- M-theory.  The five superstring theories and 11-D Supergravity can be thought of as classical limits.  Previously, we've tried to deduce their quantum theories by expanding around these classical limits using perturbation theory.  Perturbation has its limits, so by studying non-perturbative aspects of these theories using dualities, supersymmetry, etc. we've come to the conclusion that there only seems to be one unique quantum theory behind it all.  This uniqueness is very appealing, and much of the work in this field will be directed toward formulating the full quantum M-theory.
M-theory and the web of dualities

Back Next Contents Home