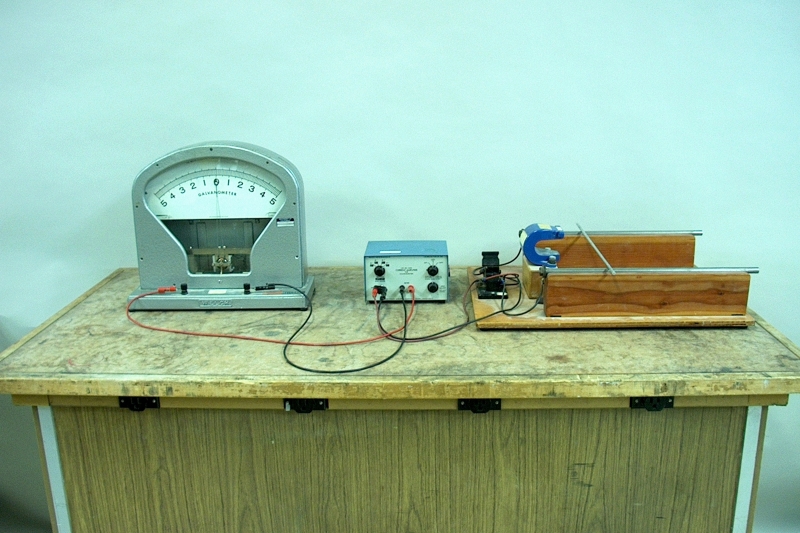
A thin metal rod lies across two larger diameter metal rods, or rails, which are connected to a galvanometer via a current amplifier. Grasp the thin rod, and, making sure to maintain good contact with the rails, rapidly slide it along them into and out of the U magnet. The needle deflection shows the generation of an EMF.
As in demonstration 72.03 -- EMF induced by moving magnet, a changing magnetic flux in a wire loop induces an electromotive force (emf) in the loop. In this demonstration, however, you change the magnetic flux by moving the rod along the rails. This is like moving a rectangular loop through a magnetic field (that is, into or out of it), or changing the size of a loop that is sitting in a magnetic field. In either case, what you are doing is changing the magnetic flux going through the area of the loop.
You can think of the rod and rails as the sides and one end of a rectangular loop of wire, the other end of which is closed by a large resistor hidden under the double-pole, double-throw knife switch behind the U magnet (and also by the input of the current amplifier). As you move the rod toward the magnet, you are continually decreasing the magnetic flux within the loop. The north pole of the magnet is on top, so the magnetic field lines point downward. If we decrease the magnetic flux, then the current from the induced emf must flow in the direction that would reinforce the magnetic field (that is, oppose the decrease in flux; see demonstration 72.09 -- Lenz’s law). From the right-hand rule (see demonstration 68.13 -- Right-hand rule model), we can see that this current must flow through the thin rod from back to front, that is, toward us as we face the apparatus as it appears in the photograph above. This makes the front rail positive with respect to the other one. With the switch flipped toward the back, as in the photograph, the front rail is connected to the positive side of the current amplifier input, and the galvanometer needle swings to the right.
When you pull the rod out of the magnet, you cause the magnetic flux to increase through the loop. To oppose this increase, the current must now flow from the front rail to the back, making the front rail negative. The galvanometer needle swings to the left.
You can also imagine how the charge carriers in the rod move as you slide it along the rails. If you slide the rod to the left, since the magnetic field points downward, qv × B points toward the front of the table, the same direction as the current in the description above. (We are considering positive current flow. You can, of course, do this for the electrons, but their negative charge flips the direction. They thus flow in the opposite direction, which agrees with our description for positive current flow.) Conversely, if you slide the rod to the right, qv × B points toward the rear of the table, again agreeing with the description above. (See any of the following: 68.21 -- Jumping wire; 68.24 -- Current-carrying rod rolls into and out of ‘U’ magnet; 68.27 -- Current-carrying aluminum foil under ‘U’ magnets; 68.33 -- Cathode ray tube, magnet; 68.34 -- Cathode ray tube, coil.)
If you wish, you can flip the DPDT switch to the front, which exchanges the connections between the rails and the positive and negative sides of the amplifier input. This, of course, reverses the direction of the galvanometer deflection with respect to the motion of the rod into and out of the magnet.
The magnetic flux through a surface is ΦB = ∫B · dS. If the magnetic field, B, is uniform and perpendicular to the surface, and the surface is finite, then ΦB = BA, where A is the area of the surface. In this demonstration, B is perpendicular to the surface formed by the rod and rails, but it varies (continuously) with distance from the ‘U’ magnet. At any point, however, it is constant over time, and varying the area of the loop by moving the rod in and out changes A, and thus ΦB, the magnetic flux, as described above. The SI unit of magnetic field is the tesla, which equals 1 N/A·m. 1 tesla also equals 1 weber/meter2. The weber, which also equals 1 V·s (= 1 N·m/A, or 1 kg·m2/A·s2), is the SI unit of magnetic flux.
References:
1) Halliday, David and Resnick, Robert. Physics, Part Two, Third Edition (New York: John Wiley and Sons, 1977), pp. 774-8.